Trimmed Mean

A trimmed mean is a method of finding a more realistic average value by getting rid of certain erratic observations. Under this method, a percentage of highest and lowest values are cut out from both the extremes before calculating the mean. This pre-calculation elimination results in a more reliable mean value.

• Trimmed mean is a statistical measure that gets rid of percentage of observations from a given list before performing mean calculations. The eradication of the values occurs from the lower and upper end of the given set of observations.
• It is also referred to as truncated mean, it provides a more reliable statistical mean value by reducing standard errors caused by the outliers or extreme variations.
• Truncated mean is applied for determining inflation rates, Olympics scores, and economic data that require negligible fluctuations.
• The lowest trimming is 0%, the same as the arithmetic mean.

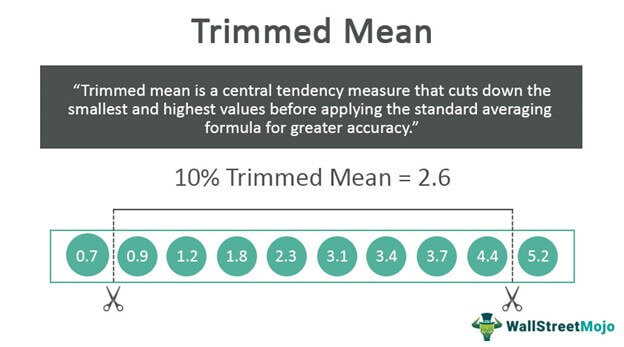
A trimmed mean is a truncated segment of the arithmetic mean used in statistics. Therefore, it is also known as the truncated mean. A percentage is cut down from both ends of the observation series, eliminating the highest and lowest values. In this series, observations are arranged in ascending order. There are three commonly applied trim percentages, i.e., 5%, 10%, and 20%. 

The truncated mean is widely applied to achieve fewer sampling fluctuations. In Olympics, the domination of extreme data points is avoided using this method. When calculating inflation rates, the truncated mean helps in excluding drastic price changes. The Reserve Bank of Australia, like many central banks, uses truncated mean values for determining inflation rates.

The rationale for the truncated mean is that there are sometimes substantial changes in prices for particular items that significantly affect a conventional average of all price changes. Sometimes, an all-inclusive mean is entirely unrepresentative of price changes of other goods and services. Trimming comes to the rescue in such cases to arrive at a more realistic value. Following are traits of certain percentages of the truncated mean.

#1 - 5% Truncated Mean

A total 5% truncated mean is usually effective in case of extensive data. 2.5% of observations are excluded from the lower and upper ends. Consequently, 95% of the observations in the series remain intact. A 5% truncated self would reflect applying separate 5% trim on the lowest and highest values. By that logic, it is crucial to understand that with a 0% trim, one would not be eliminating any values and will perform mean by taking into account each observation. As such, it would result in the arithmetic mean.

#2 - 10% Truncated Mean

A total of 10% truncated mean is used very often. In the Olympics, this method is often employed to eradicate the traditional mean biases caused by extreme values. So, 5% of the smallest and highest values are removed before calculating the mean from each end. Consequently, 90% of the observations in the series remain intact.

#3 - 20% Truncated Mean

A 20% truncation is similar to other trim percentages. Here, 10% of the values are removed from both the ends. Consequently, the remnant is used to calculate a 20% truncated mean. Additionally, a 5,10, or 20 percent cutting down from a suggested end of the list will require one to perform the calculation on only the given part. Therefore, a 10% truncated for the highest value would require only removing observations from amongst the higher end.

The following steps are involved in a trimmed mean formula –

1. Go through the percentage of trimming. It could be 10%, 20%, 30%, etc.
2. Multiply the percentage by the number of observations to arrive at the number of values deducted from each end.
3. Remove the highest and lowest numbers from both ends.
4. Reduce the total number of observations by deducting the number of observations that were cut.
5. Apply standard mean or average formula to the remaining observations, which is as follows - Sum of observations/Number of observations
6. After applying the average formula, one would arrive at the truncated mean.

The following numerical problem shows the manual application of the truncated mean concept and the step-by-step calculations involved.

Problem:

A shot-put player records the following scores, 16.8 m, 16.9 m, 17.1 m, 17.2 m, 17.8 m, 17.9 m, 18.2 m, 18.3 m, 18.3 m, 18.5 m. Find the 10% trimmed mean.

Solution:

Given below is the process of computing the truncated mean:

Step #1 - Count the number of observations, i.e.,

No. of observations = 10

Step #2 - Arrange the series of observations in ascending order, i.e., from the smallest to the highest value.

Scores arranged in ascending order:

16.8 m, 16.9 m, 17.1 m, 17.2 m, 17.8 m, 17.9 m, 18.2 m, 18.3 m, 18.3 m, 18.5 m

Step #3 - Figure out the percentage to be cut on each end of the observation series.

Percentage = 10%

Number of observations to be cut from both ends = 10% * 10 = 1

As such, we’d need to remove two numbers from the set of observations.

Step #4 - Let’s remove the highest and lowest number from both ends. It gives us

16.9 m, 17.1 m, 17.2 m, 17.8 m, 17.9 m, 18.2 m, 18.3 m, 18.3 m,

Step #5 - Let us apply the standard mean or average formula to calculate the mean from the remaining eight values.

16.9, 17.1, 17.2, 17.8, 17.9, 18.2, 18.3, 18.3 / 8

Trimmed mean = 17.71

For real-world applications, the determination of the percentage to be cut is crucial. Experience, knowledge, and practice help users decide a suitable percentage that has to be cut out from the arithmetic mean. This percentage varies depending on the situation, problem, and niche where it is applied.

This has been a guide to Trimmed Mean and its statistical definition. Here we discuss the example with step-by-step calculation along with formula. You may learn more about financing from the following articles –

• Geometric Mean
• Harmonic Mean Formula
• Population Mean Formula
• Weighted Mean Formula