Standard Deviation

Last Updated :

Blog Author :

Edited by :

Reviewed by :

Table Of Contents

Standard Deviation Definition

Standard deviation measures the amount of variation or dispersion in a set of data values relative to its mean (average). It is a statistical tool used for interpreting the reliability of data. It is represented by the symbol ‘σ.’

If the deviation is less, data points are close to the mean value, and data is considered
reliable. In contrast, if the deviation is wide, data points are spread farther from the mean value; such data is considered less reliable. Standard deviation is used in the analysis of overall risk and returns pertaining to a portfolio.

Standard Deviation Definition

A Standard deviation is a statistical tool that measures the volatility of data. It indicates the extent to which sample values deviate from mean values. It is computed as the square root of the variance and represented by the symbol' σ' (Greek letter).
The σ cannot be a negative value, and it can only be 0 if the values in a data set are equal and have nil variation.
In finance, this mathematical tool is applied to identify the level of risks involved in a particular investment or asset. The method measures the spread of respective prices and returns. Higher deviation reflects high volatility and vice-versa.
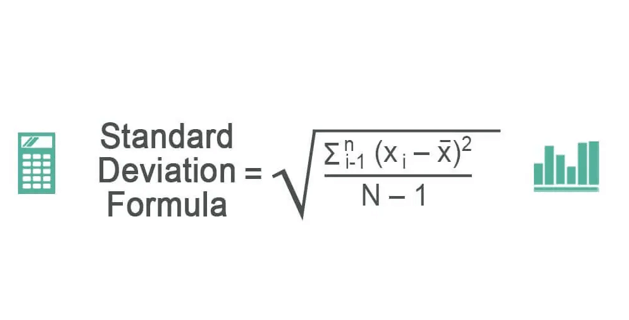
If the symbol σ denotes standard deviation, n is the total number of observations in a data set, xi is the ith number of observations, and µ is the sample mean, then deviation is computed by the following formula:

Standard Deviation Explained

Standard deviation is a measure of volatility in data distribution relative to mean values. It is a statistical tool applied in business, finance, and investment to evaluate the risk profile of assets.

In most cases, a minimal standard deviation is considered favorable. If the deviation in previous price fluctuations is low for a particular stock, it is considered a secured investment opportunity.

This statistical tool helps researchers and analysts understand the spread of data—to
determine the extent to which data is scattered. This mathematical tool indicates the
dispersion of sample values from the mean value.

Standard errors highlight the sample mean's accuracy relative to the population
mean—when data is vast and widely spread. On a graph, the deviation can lie to the left, to the right, or both—forming a Bell-Shaped Curve.

Standard Deviation Equation

The equation for determining the standard deviation of a series of data is as follows:

SD-Formula-3
i.e, σ=√v

Also, µ =∑x/n

Here,

σ is the symbol that denotes standard deviation.
n is the number of observations in a data set.
x_i is the i^th number of observations in the data set.
µ is the mean of the sample.
V is the variance.
∑x is the sum of all values in a data set.

Calculation

The basic steps used to find and calculate standard deviation are as follows:

First, determine the mean of the data set.
Next, prepare a chart listing sample values and the difference between sample
values and mean values.
In the next column, find the square of the differences.
To obtain the variance, add all the squares and divide the result by the difference between the total number of observations and 1.
Finally, find the square root of the variance to derive the standard deviation.

Example

Let us go through some examples to understand the practical implications:

Find the deviation in crude oil prices in a year when average monthly prices per liter were as follows:

Month	Average Price Per Litre in $
January	0.83
February	0.81
March	0.78
April	0.82
May	0.79
June	0.75
July	0.76
August	0.79
September	0.81
October	0.77
November	0.76
December	0.75

Solution:

Calculation of Mean:

µ = ∑x/n

µ = 9.42/12

= $0.785 per liter

S. No	Month	Average Price Per Liter in $ (x)	x - $0.785	(x - $0.785)²
1	January	0.83	0.045	0.002025
2	February	0.81	0.025	0.000625
3	March	0.78	-0.005	0.000025
4	April	0.82	0.035	0.001225
5	May	0.79	0.005	0.000025
6	June	0.75	-0.035	0.001225
7	July	0.76	-0.025	0.000625
8	August	0.79	0.005	0.000025
9	September	0.81	0.025	0.000625
10	October	0.77	-0.015	0.000225
11	November	0.76	-0.025	0.000625
12	December	0.75	-0.035	0.001225
12	-	9.42		0.0085

Calculation of Standard Deviation :

SD Formula

σ = √
σ = √ (0.00077272727)
σ = $0.0277979724571285

Thus, the standard deviation in crude oil prices per liter for the given year is
0.0277979724571285.

Interpretation

Standard Deviation indicates volatility or dispersion in the values of a particular distribution. It depicts the extent to which sample values deviate from mean values. Thus, this measure facilitates comparison and analysis.

Following are the various interpretations of the acquired result:

If σ is high, then the volatility of the analyzed data is also high.
Similarly, when σ is low, the dispersion between data points is also meager.
In a distribution, σ can be 0 only when the difference between data points is nil. It is also the smallest value of deviation one can get.
It is impossible to derive a negative value of σ since the numerator includes the square of the differences between sample values and mean values.
Also, the number of observations is always more than 1; therefore, the denominator has to be a positive value.
The standard deviation is measured in the same unit as the distribution values. For instance, in the above example, σ is expressed in $.
The outliers (extremely high or low values) significantly impact deviation measurements.

Frequently Asked Questions (FAQs)

What is a standard deviation?

Standard Deviation is a statistical method used for finding the spread of data in distribution using mean values. It is denoted by the symbol 'σ.'

How do you calculate standard deviation?

Standard deviation is calculated as the square root of variance. Variance is the summation of the square of the difference between each value in the data set and their mean values, divided by the value acquired by subtracting one from the total number of observations.

Why is standard deviation important?

It identifies the degree of variability among the values in a sample distribution. It is a widely used statistical tool in finance, investment, and business for interpreting the magnitude of risk involved in a security or asset. In most cases, a minimal deviation is considered favorable. If the deviation in previous price fluctuations is low for a particular stock, it is considered a secured investment opportunity.

Can the standard deviation be zero?

The only case where it can be zero is when all the data points in distribution are the same. A zero deviation indicates nil scattering or variability among values. For real-world scenarios, that is nearly impossible.