Table Of Contents
Simple Savings Calculator
This simple savings calculator can be used to calculate what will the total value of investment done by the investor over a period of time.
About Savings Calculator
The formula is per below:
P*(1+r)n + I*
Wherein,
- P is the Initial amount invested
- I is the periodically equal savings invested
- r is the rate of interest per annum
- n is the number of period or frequency wherein the amount is to be invested
It can be used to calculate the future value of the investment amount where the investor invested a lump sum amount and, thereafter, invests a smaller equal amount periodically as per his convenience. This calculator can be used where an investor invests either in recurring fixed deposits or in mutual funds or any other product where the investor is required to invest in equal installments with equal amounts. This can help the investor decide where to invest in and which product to select and what amount will be due to him at the end of the investment period or, in other words, at the time of maturity.
How to Calculate Using Savings Calculator?
One needs to follow the below steps in order to calculate the investment maturity value.
First of all, determine the initial amount that is to be invested as a lumpsum amount.
Now, Compound the initial amount either monthly, quarterly, semi-annually, or annually by the rate of interest until the maturity period as the case may be.
We now need to determine the future value of the monthly installment amount with the same rate of interest that was used to calculate the maturity value of the initial investment.
- Now, we can take a total of values arrived on step 3 and step 4, which shall be the savings maturity value.
Example #1
Mr. Winter is a newbie in the investment field and wants to invest in the stock market. However, he does not want to take the risk. He approaches a financial advisor, and he gets confused with the term he uses; and in his final discussion, the advisor tells him to learn about the markets first and start investing in mutual funds. Since he was sitting with idle cash, the financial advisor advised him to invest $5,000 as a lump sum in a debt scheme and invest $100 monthly for 3 years to learn about the market and see how the investment grows. On average, the debt scheme in which he will be investing earns 7.5% p.a.
Based on the given information, you are required to calculate what would be the value of an investment after 3 years, assuming that the investment takes place at the end of the period?
Solution:
We need to calculate the maturity value of the initial investment, which is $5,000 here, and along with it, we need to calculate what will be the future value of the monthly savings that are invested in this debt scheme, which is $100, and the term is 3 years which is 36 months.
The interest earned on the investment is 7.5%, and when it compounds monthly, it shall be 7.5%/12, which is 0.63%.
| Sr No | Particulars | Amount |
|---|---|---|
| 1 | Initial Amount | $5,000.00 |
| 2 | Loan % | 100% |
| 3 | Equal Amount Savings | $100.00 |
| 4 | Length of Investment | 3 |
| 5 | Rate of Interest per Annum | 7.5% |
| 6 | Frequency of Installment | 12 |
| 7 | Total Number of Payments | 36 |
| 8 | Monthly Interest Rate | 0.63% |
We can now use the below formula to calculate the savings total.
Installment = P * (1+r)n + I *

= $5,000 x (1+0.63%)36 + $100 x
= $10,280.37
Hence, the maturity value will be $10,280.37
Example #2
Mrs. Kavita, aged 57 years, is nearing retirement from the firm where she has worked for around 20 years. She has now become a risk-averse person and wants to lead a safe life now wherein she gets a quarterly fixed amount for her spending. She is interested in investing in a fixed deposit scheme where she will deposit $56,000 as initial, and then she would be depositing quarterly $2,000 until the next 3 years so that after she retires, she has the lump sum amount, which then she will use to invest in quarterly paying out interest fixed deposit scheme. The current rate of interest is 8%.
Based on the given information, you are required to calculate the savings she would have at the time of retirement.
Solution:
We need to calculate the maturity value of the initial investment, which is $56,000 here, and along with it, we need to calculate what will be the future value of the quarterly savings that are invested in this fixed deposit scheme, which is $2,000, and the term is 3 years which is 12 quarters.
The interest earned on the investment is 8.00%, and when it compounds quarterly, it shall be 8.00%/4, which is 2.00%.
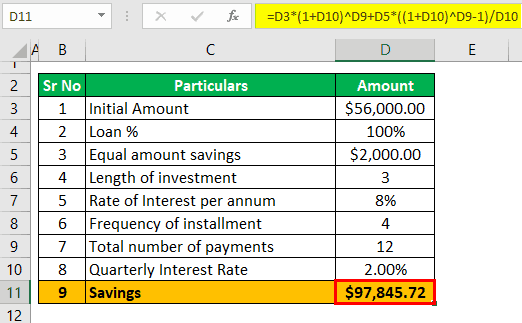
| Sr No | Particulars | Amount |
|---|---|---|
| 1 | Initial Amount | $56,000.00 |
| 2 | Loan % | 100% |
| 3 | Equal Amount Savings | $2000.00 |
| 4 | Length of Investment | 3 |
| 5 | Rate of Interest per Annum | 8% |
| 6 | Frequency of Installment | 4 |
| 7 | Total Number of Payments | 12 |
| 8 | Quarterly Interest Rate | 2.00% |
We can now use the below formula to calculate the savings total.
Savings = P*(1+r)n + I *

=$56,000 x (1+2.00%)12 + $2,000 x
=$97,845.72
Hence, the maturity value will be $97,845.72.
Conclusion
The savings calculator, as discussed, can be used to calculate the maturity value of the investment, which is done in periodical installments and as well as by investing a certain amount as lump sum. The rate of interest earned could be monthly, quarterly, semi-annually, or annually.