Sampling Distribution Formula

What is the Sampling Distribution Formula?

A sampling distribution can be defined as the probability-based distribution of particular statistics and its formula helps in calculation of means, Range, standard deviation and variance for the undertaken sample. Since sample distributions are derived from several samples it is not necessary that the graphs of such distribution would be in the form of a bell shape. Preparations of sampling distribution help researchers, marketers, statisticians pursue their research work.

The formulation of correct sample distribution helps in the effective preparation of hypothesis analysis and determination of model score. In sampling distribution, samples are normally very large. It could also consist of a sample that is composed of only average values.

The probability distribution is basically sampling distributions itself and it is employed to determine the variance or standard deviation of the sample statistics. The formula for sampling distributions is determined by using the central limit theorem. As per the theorem, for any random sample having size n arranged from random distribution would have a sample mean and sample variance which would normal to the population mean.

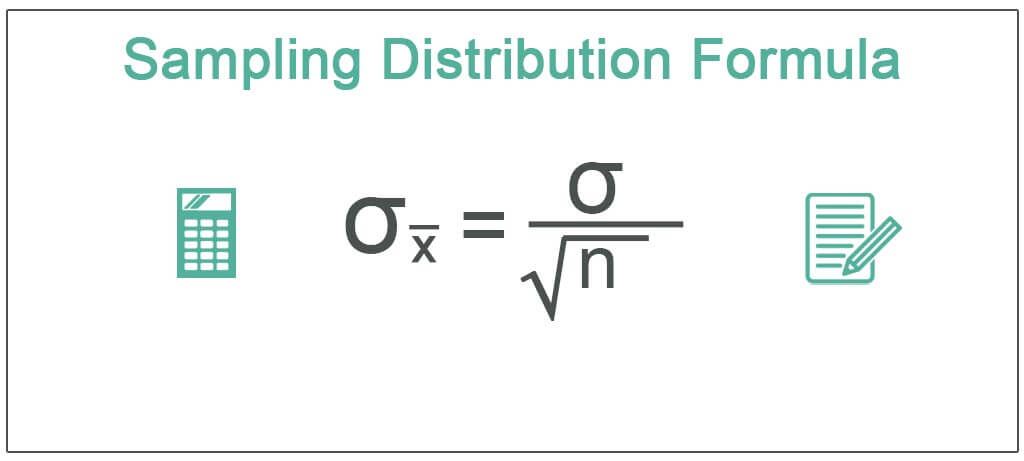
For a sample size of more than 30, µ͞x =µ and σ_͞x =σ / √n

Here,

The mean of the sample and population are represented by µ͞x and µ.
The standard deviation of the sample and population is represented as σ_͞x and σ.
The sample size of more than 30 represents as n.

Explanation

The formula for Sampling Distribution can be calculated by using the following steps:

Step 1: Firstly, find the count of the sample having a similar size of n from the bigger population of having the value of N.

Step 2: Next, segregate the samples in the form of a list and determine the mean of each sample.

Step 3: Next, prepare the frequency distribution of the sample mean as determined in step 2.

Step 4: Next, determine the probability distribution of the determined sample means after determining the frequency distribution in step 3.

Examples of Sampling Distribution Formula (with Excel Template)

Let’s see some simple to advanced practical examples of the sampling distribution equation to understand it better.

You can download this Sampling Distribution Formula Excel Template here – Sampling Distribution Formula Excel Template

Example #1

Let us take the example of the female population. The size of the sample is at 100 with a mean weight of 65 kgs and a standard deviation of 20 kg. Help the researcher determine the mean and standard deviation of the sample size of 100 females.

Popular Course in this category

Financial Modeling Course (with 15+ Projects)4.9 (927 ratings) 16 Courses | 15+ Projects | 90+ Hours | Full Lifetime Access | Certificate of Completion

View Course

Solution

Use below given data for the calculation of sampling distribution

The mean of the sample is equivalent to the mean of the population since the sample size is more than 30.

Calculation of standard deviation of the sample size is as follows,

=20/√100

Standard Deviation of Sample Size will be –

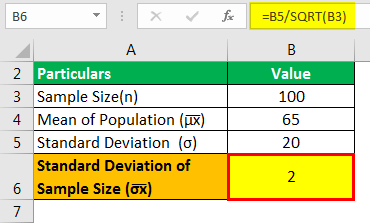
σ_͞x =2

Therefore, the standard deviation of the sample is 2 and the mean of the sample is at 65 kg.

Example #2

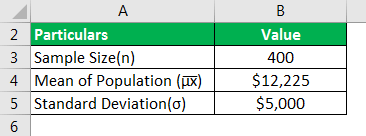
Let us take the example of taxes paid by the vehicles. In the state of California, the average tax paid is $12,225 having a standard deviation of $5,000. Such observations were made on the sample size of 400 trucks and trailers combined. Help the transport department to determine the mean and standard deviation of the sample.

Solution

Use below given data for the calculation of sampling distribution

Calculation of standard deviation of the sample size is as follows,

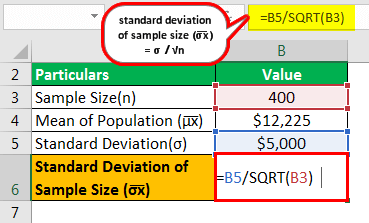
= $5,000 / √400

Standard Deviation of Sample Size will be –

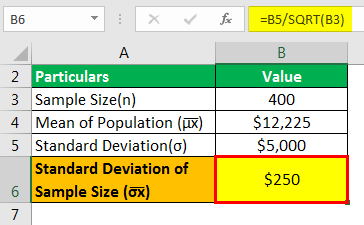
σ_͞x =$250

Therefore, the standard deviation of the sample as assessed by the department of transport is $250 and the mean of the sample is $12,225.

Example #3

Let us take the example of the following data is displayed below:

Help the researcher determine the mean and standard deviation of the sample.

Determine the mean of the sample as displayed below: –

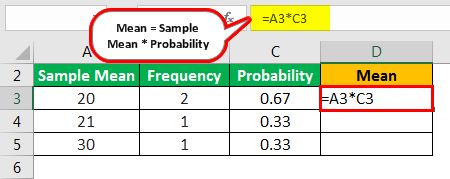
=20*0.67

Mean will be –

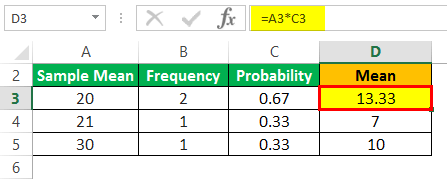
=13.33

Total Mean

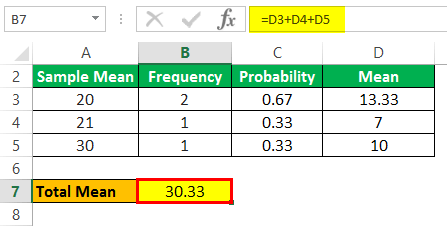
=13.33+7+10
Total Mean =30.33

Determine the variance of the sample as displayed below: –

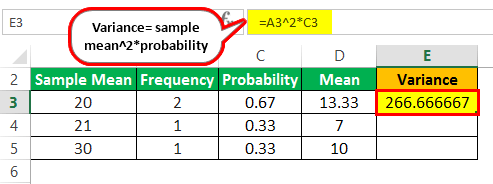
=20^2*0.67
=266.66667

Variance

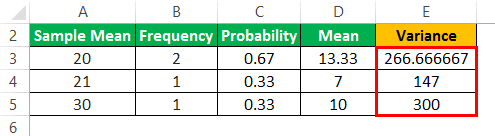
Total Variance

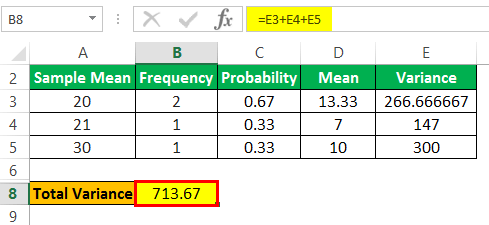
= 713.67

Calculation of standard deviation of the sample size is as follows,

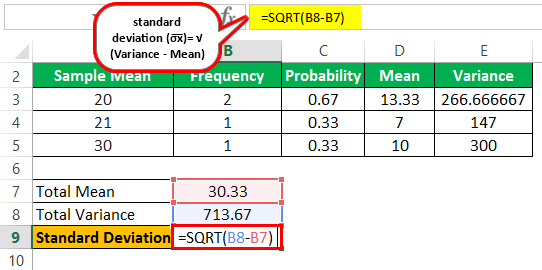
σ_͞x = √ 713.67 – 30.33

Standard Deviation will be –

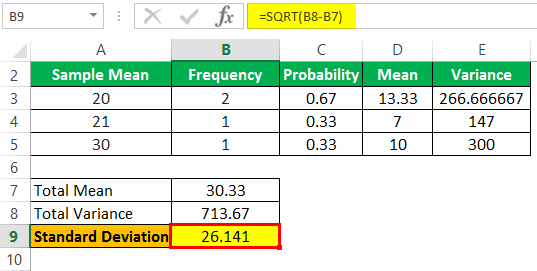
σ_͞x = 26.141

Therefore, the standard deviation of the sample as assessed by the researcher is 26.141 and the mean of the sample is at 30.33.

Relevance and Use

The sampling distribution is utilized by many entities for the purpose of research. It could be analysts, researchers, and statisticians. Whenever population size is large, such methodology helps in formulations of the smaller sample which could then be utilized to determine average means and standard deviations. The average means can be plotted on the graph to arrive at the uniform distribution relating to the population and if the researcher increases the sample size, the probability of graph reaching normal distribution enhances.

It helps in major simplification of the inferences taken up in statistics. It further helps in deducing analytical contemplation by determining the frequency of the probability distribution of sample means. The sampling distribution forms base for several statistical concepts that may be used by the researchers to facilitate their hypothesis.

What is the Sampling Distribution Formula?