Rule Of 70

The term “Rule of 70'' or also known as doubling time, refers to the total time required to double the quantity or value (we have taken money). It simply means that if all other factors remain constant, then in how much time it will take to double our money or investments or profit.

For instance, suppose if we have invested $100 at a growth rate of 5% per annum, then in how much time our money invested has doubled, i.e., becomes $200. The above-said method is also known as doubling time or in simple time required to double the amount.

• The rule of 70 is a mathematical approximation used to estimate the time it takes for a quantity to double in exponential growth or compound interest scenarios.
• The rule of 70 states that if a quantity grows at a constant annual rate, it will approximately double in size after about 70 divided by the growth rate.
• The rule of 70 is derived from the mathematical constant e, which is the base of the natural logarithm.
• The rule of 70 is widely used in finance, economics, demography, and other fields to estimate doubling times and understand the pace of growth or compounding.

The concept of rule of 70 explains within what time an investment will achieve double of its original amount. This is a common metod used in the financial market to analyse and compare the returns of investments that have separate annual rates.

This approach of rule of 70 equation is very quick and easy to implement and helps in calculating an approximate time during which the amount will become double. However, the growth rate or the rate of return is assumed to remain constant. It smain purpose is time estimation, which is a significant factor when making investment-related decisions. This is because every investor has a time horizon and expects to get the desired return within that period. It helps in planning for various events or contingencies and ensures financial strength and support.  

It is a straightforward and convenient approach, assisting in future planning and estimation. Analysts and investors often use this tool to make projections or evaluations without resorting to complex calculations and models, even though it provides an estimation, not accurate data. In case the investor’s time horizon is less than the number of years calculated in the rule, they can make changes to their portfolio and adjust it to make it suitable for their requirement.

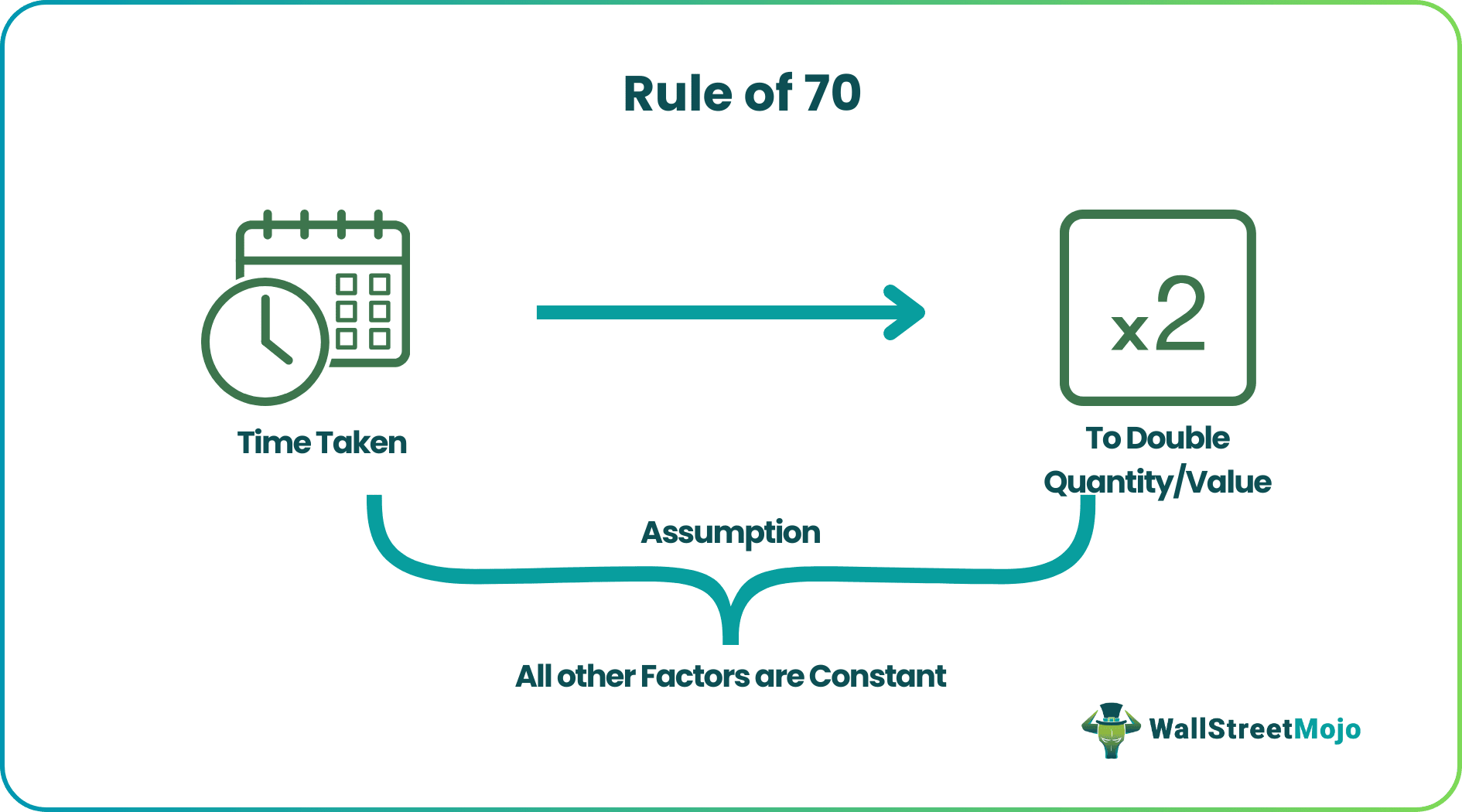
In this article, we will focus on the formula and rule of 70 equation for calculating the Doubling time using the rule of 70, which is expressed as the division of 70 by the % of growth rate. Mathematically, it is represented as:

For the calculation of growth rate, we need to apply the following formula:

Growth Rate (per annum) = (Amount Received on maturity subtracted by Amount Invested) divided by Amount invested thereafter multiplied by 365 days / 12 months divided by period of investment. Mathematically it can be represented as:

Let us look at the steps to calculate the time using the above formula of rule of 70 in economics.

The equation for Rule of 70 can be derived by using the following steps:

Step 1: Firstly, determine the number of investments and the period of investment.

Step 2: Then, calculate the return on investment, which we got by subtracting the amount invested from the amount received on maturity called “Return."

Step 3: Then, determine the period of investment in which we got returns or capital appreciations, i.e., the period from the date of investment to maturity called “T."

Step 4: By using the above-mentioned formula for calculating the growth rate, calculate the growth rate of the investment called “G."

Step 5: Finally, by using the above-mentioned formula, we can easily calculate Doubling Time. Doubling time, as calculated in step 4.

Let’s see some simple to advanced examples to understand rule of 70 in economics better.

Example #1

Suppose Mr. A has invested $100000 on the first day of the month in equity-oriented funds for a period of 3 months. At the time of maturity, Mr. A has received a sum of $150000. Now Mr. A wants to know the doubling time assuming all other factors in which business operates remain constant.

Solution:

Use the given data for the calculation of the rule of 70.

Then for calculation of growth rate, the following steps are to be taken:

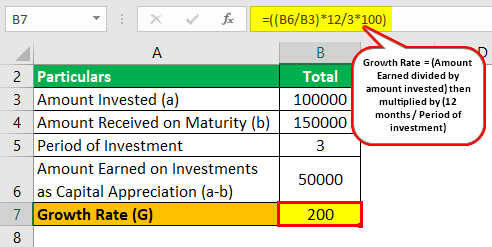
Amount Earned on Investments = Amount Received on Maturity – Amount Invested

• Amount Earned on Investments = $(150000-100000) = $50000.
• Growth Rate = ((50000/100000) * (12/3) * 100)
• Growth Rate = 200% per annum

Now, Calculation of Doubling Time can be done as follows,

• Doubling Time = 70 / 200

Doubling Time will be -

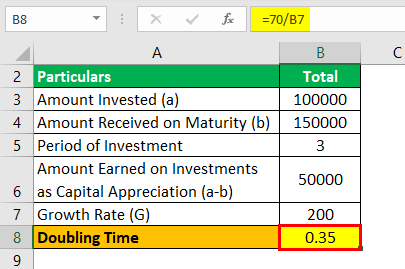
• Doubling Time = 0.35 years.

Example #2

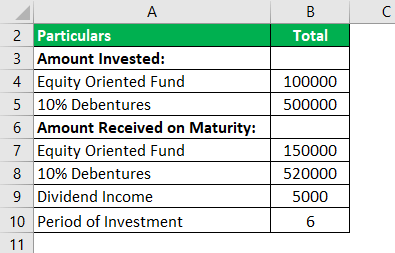
Suppose Mr. A has invested $100000 on the first day of the month in equity-oriented funds and has earned a dividend on these investments amounting to $5000. He has also invested in 10% debentures of PQR Inc., amounting to $500000. The period of both investments is 6 months. At the time of maturity, Mr. A has received a sum of $150000 from the equity-oriented fund and $520000 from 10% debentures. Now Mr. A wants to know the doubling time assuming all other factors in which business operates remain constant, and PQR Inc. pays interest on a half-yearly basis.

Solution:

Use the given data for the calculation of the rule of 70.

Amount Earned on Investments = Amount Received on Maturity – Amount Invested

• On Equity fund = $(150000-100000) = $50000.
• On 10% Debentures = $(520000-500000) = $20000

Other incomes earned from investments:

• Dividend Income = $5000
• Interest Income on 10% Debentures = $500000*10%*6/12 = $25000
• Total other income and capital appreciations = $(50000+20000+5000+25000) = $100000.

Growth rate (G) can be calculated by using the above formula:

• Growth Rate = $(100000/600000) * (12/6)*100
• Growing Rate = 33.33% per annum

Calculation of Doubling Time can be done as follows,

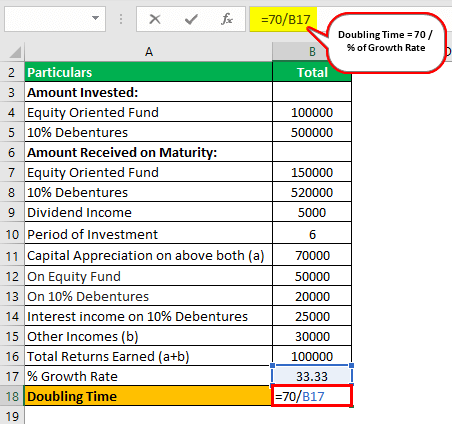
• Doubling Time = 70 / 33.33

Doubling Time will be -

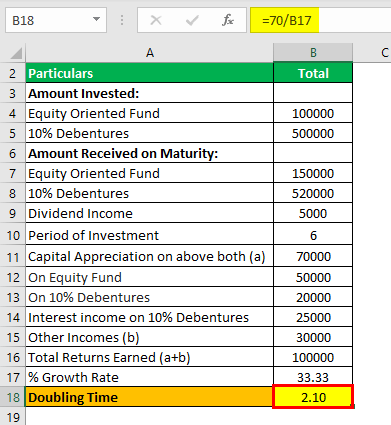
• Doubling Time = 2.10 years.

The above examples explain clearly how to use the method of rule of 70 macroeconomics to calculate the doubling time of an investment under different scenarios. It helps us to understand how to identify financial data and use them to calculate the time and accordingly take investment related decisions.

You can use this rule of 70 calculators to calculate rule of 70 macroeconomics.

This calculation is useful not only in finance but also in various other fronts. Let us find out the areas where it is widely used or has the prospect of usage.

• By applying the rule of 70 or the Doubling time concept, a short-term investor or anyone may get to know the time required to double the money invested, assuming all other factors remain constant such as growth rate, etc.

• It helps to determine the duration of investments. In other words, it helps investors to get the rough idea of “In how much time an investor got its money doubled” and for how long they have to keep investing the money.
• The divisibility rule of 70 can be used at the macroeconomic level to estimate ethe time a country can take to double it Gross Domestic Product (GDP) or its population growth. This is extremely useful for economic and financial planning, growth, expansion, investment, trade and commerce, etc.
• Corporates and businesses widely use it to make business planning, sales and growth projections, market expansion plans, new product planning and investment for the sale, revenue target and boosting profitability, etc.
• This is a concept with helps in communicating how fast the growth or decay of money can take place.
• Students who aspire to learn more about investments and finances can use the divisibility rule of 70 to expand their knowledge.
• Since it is not a complex concept, it can be calculated quickly, and any person can use it since it is easy to understand.

Thus, the above are common areas where this process can be successfully implemented and used. It  is a valuable and handy concept for financial and economic planning.

Both the methods are used for quick estimation and particularly used in the field of finance to calculate the approximate time of doubling an amount.

However, the difference between them are as follows:

The former uses 70 divided by the annual growth rate to get the time within which the investment will double and the latter uses 72 and divides it by the annual growth rate to get the same the time it will take for the investment to double. Both are similar approaches with a different numerator.

This has been a guide to what is the Rule Of 70. We explain the formula, along with calculator, examples, differences with rule of 72 & its uses. You can learn more about financial analysis from the following articles –

• Dividend Aristocrats
• Rule of 72
• Compounding Quarterly
• Compounding Calculations