Portfolio Return Formula

Publication Date :

03 Nov, 2023

Blog Author :

Edited by :

Reviewed by :

Table Of Contents

What Is The Portfolio Return Formula?

Portfolio Return Formula refers to the mathematical equation used in order to calculate the return of the total portfolio consisting of the different individual assets where according to the formula portfolio return is calculated by calculating return on investment earned on individual assets multiplied with their respective weight class in the total portfolio and adding all the results together.

Portfolio return can be defined as the sum of the product of investment returns earned on the individual asset with the weight class of that individual asset in the entire portfolio. It represents a return on the portfolio and just not on an individual asset.

What Is The Portfolio Return Formula?

The portfolio return formula calculates the overall return of a portfolio by considering the weight of each investment and their respective returns.
Multiply the weight of each investment by its return and sum up these weighted returns to calculate the portfolio return.
The formula enables investors to evaluate the performance of their investment portfolio and compare it to benchmarks or targets.
It is important to note that the portfolio return formula assumes proportional weighting and does not account for factors like transaction costs or taxes.

Portfolio Return Formula Explained

Portfolio Return Formula helps calculate the portfolio return to help investors learn about the profits or losses gained or incurred as a result of investing in different instruments. Based on the expected inputs, one can calculate the returns and, in turn, and plan their expenses or cut them short. In addition, it guides investors to assess whether their investments would be fruitful if they apply similar strategies in the future.

Investing in a portfolio involves investors spending on different types of assets and instruments at the same time. In short, a collection of investments is a portfolio. Such investments help one have a diversified investor profile. With these investments, investors look for balanced returns, which means if one instrument incurs loss, the other would make up for it by reaping profits. The portfolio return, therefore, needs to generate a balanced figure for investors for them to keep investing.

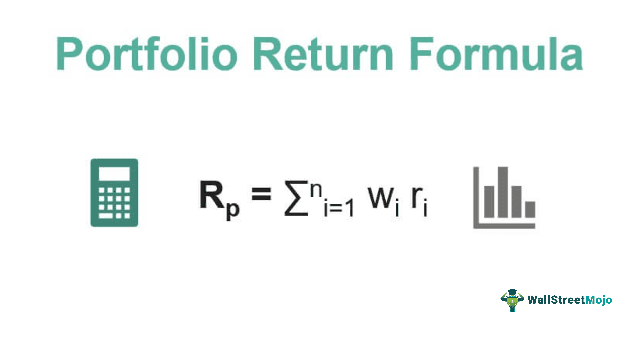
The expected return can be calculated with a product of potential outcomes (i.e., returns which is represented by r in below) by the weights of each asset in the portfolio (i.e., represented by w), and after that calculating the sum of those results.

R_p = ∑ⁿ_i=1 w_i r_i

Where ∑ⁿ_i=1 w_i = 1

w is the weight of each asset
r is the return of an asset

How To Calculate? (Step by Step)

The calculation of the portfolio return is quite simple but requires little attention.

Get the individual asset return in which the funds have been invested in. For example, if an investor has invested in equity, then one needs to calculate the entire return, that is total return, including the interim cash flows, which, in the case of equities, would be a dividend .
Calculate the weights of the individual asset in which funds are invested. This can be done by dividing the invested amount of that asset by total fund invested.
Take the product of return that is calculated in step 1 with weight, which is calculated in step 2.
The third step will be repeated until the calculations of all the assets are completed. Then finally, we need to add up the product of all the individual asset returns by its weight class, which shall be the portfolio return.

Examples

Let us consider the following instances to understand the calculation of portfolio return formula in Excel:

Example #1

Consider ABC Ltd, an asset management company, has invested in 2 different assets along with their return earned last year. You are required to earn a portfolio return.

Solution:

We are given the individual asset return and along with that investment amount, therefore first we will find out the weights as follows,

Weight (Asset Class 1) = 1,00,000.00 / 1,50,000.00 =0.67

Similarly, we have calculated the weight of Asset Class 2

Weight (Asset Class 1) = 50,000.00 / 1,50,000.00 =0.33

Now for the calculation of portfolio return, we need to multiply weights with the return of the asset, and then we will sum up those returns.

W_iR_i (Asset Class 1) = 0.67*10% =6.67%

similarly, we have calculated W_iR_i for Asset class 2

W_iR_i (Asset Class 2) = 0.33*11%
=3.67%

Calculation of portfolio return is as follows,

Portfolio Return

The portfolio return will be 10.33%

Example #2

JP Morgan chase, one of the largest investment banking firms, has made several investments in various asset classes. Mr. Dimon, the company chairman, is interested in knowing the returns on the overall investment done by the firm. You are required to calculate the Portfolio Return.

Solution:

We are here only given the latest market value, and there are no returns given directly. Hence, first, we need to calculate return on individual assets.

We need to subtract the investment amount from market value to arrive at excess return, and then dividing the same by investment amount will yield our returns on the individual asset.

Note: For detailed calculation, please refer to the excel template.

We now have the individual asset return and along with that investment amount and now we will find out the weights using investment amount and not the market value as follows,

Weight of Equities = 300000000 / 756500000 = 0.3966

Similarly, we have calculated the weight of all the other particulars.

Now for the calculation of portfolio return, we need to multiply weights with the return of the asset, and then we will sum up those returns.

Calculation of portfolio return is as follows,

Portfolio Return

Hence the portfolio return earned by JP Morgan is 21.57%

Example #3

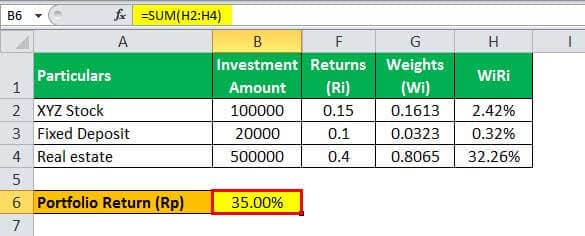
Gautam is an individual who has recently started investing in the market. He has invested in XYZ stock for 100,000, and it has been a year and since then, he has received a dividend of 5,000, and the current market value of XYZ stock is trading at a premium of 10%. Also, he has invested in a fixed deposit for 20,000, and the Bank provides a 7% return on it. And lastly, he has invested in land in his hometown for 500,000, and the current market value is 700,000. He has approached you to calculate portfolio return.

Solution:

We are here only given the latest market value, and there are no returns given directly. Hence, first, we need to calculate return on individual assets.

We need to subtract the investment amount from market value to arrive at excess return, and then dividing the same by investment amount will yield our returns on the individual asset.

Note: For detailed Calculations, please refer to the excel template.

We now have the individual asset return and along with that investment amount, and now we will find out the weights using investment amount and not the market value.

Weight (XYZ Stock) = 1,00,000 / 6,20,000 = 0.1613

Similarly, we have calculated the weight for other particulars as well.

Now for the calculation of portfolio return, we need to multiply weights with the return of the asset, and then we will sum up those returns.

(XYZ Stock) W_iR_i = 0.15 * 0.1613 = 2.42%

Similarly, we calculated W_iR_i for other particular as well.

Calculation of portfolio return is as follows,

Portfolio Return

Hence the portfolio return earned by Mr. Gautam is 35.00%

Relevance and Use

It is crucial to understand the concept of the portfolio’s expected return formula as the same will be used by those investors so that they can anticipate the gain or the loss that can happen on the funds that are invested by them. Based on that expected return formula, an investor can make a decision as to invest in an asset given their probable returns.

Further, an investor will also be able to decide on the asset’s weight in a portfolio i.e., what proportion of the funds should be invested and then do the required change.

Also, an investor can make use of the expected return formula for ranking the individual asset and further eventually can invest the funds per the ranking and then finally include them in his portfolio. In other words, he would increase the weight of that asset class whose expected return is higher.

Frequently Asked Questions (FAQs)

1. How to incorporate the effect of compounding in portfolio return calculations?

To incorporate the effect of compounding in portfolio return calculations, one can use the compound interest formula. Instead of simply summing the returns, you can calculate the compounded return by multiplying the individual returns of the assets in the portfolio. This accounts for the reinvestment of returns over time, resulting in a more accurate representation of the actual growth or decline of the portfolio.

2. Can the portfolio return be negative?

Yes, the portfolio return can be negative. A negative portfolio return indicates a loss or decline in the portfolio's value over a given period. It can occur when the combined returns of the assets in the portfolio are negative or when the losses outweigh the gains.

3. What are the limitations of the portfolio return formula?

The portfolio return formula has certain limitations. For example, it assumes that the returns of individual assets are independent and identically distributed, which may not always hold true in real-world scenarios. Additionally, it does not account for other factors, such as taxes, fees, and transaction costs, which can impact the overall portfolio returns. The formula also assumes a static portfolio composition, whereas, in practice, investors may adjust their asset allocations regularly.