What is Option Adjusted Spread?
Option-Adjusted Spread (OAS) is a yield spread which is added to the benchmark yield curve to price security with an embedded option. This spread measures the deviation of the security’s performance from the benchmark on the back of an embedded option. It is helpful in determining the price of complicated securities like mortgage-backed securities (MBS), collateralized debt obligationsCollateralized Debt ObligationsCollateralized debt obligation (CDO) refers to a finance product offered by the banks to the institutional investors. Such tranches have a complex structure and derive their value from the various underlying assets like loans, mortgages and corporate bonds, which also serve as collaterals in case of default.read more (CDO), convertible debentures, and option-embedded bonds.
The formula of Option Adjusted Spread
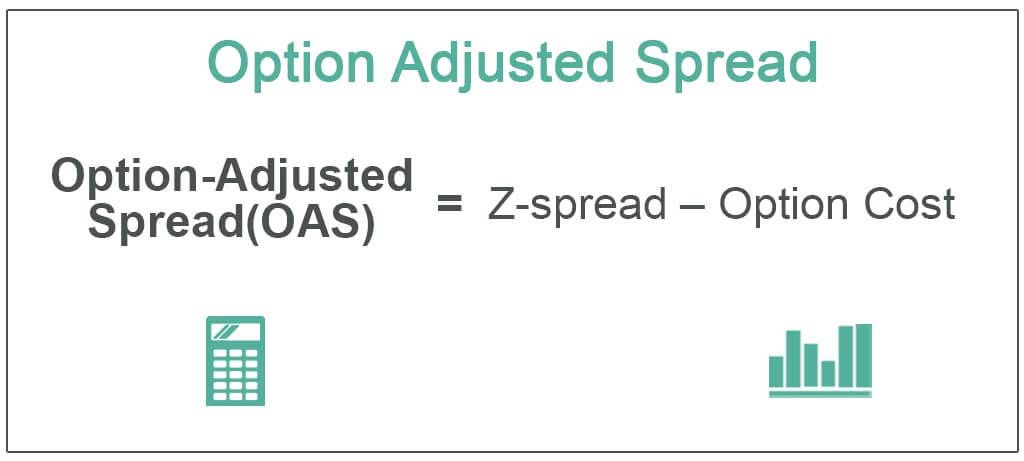
Spread differs from OAS only to the tune of options cost.
You are free to use this image on your website, templates etc, Please provide us with an attribution linkHow to Provide Attribution?Article Link to be Hyperlinked
For eg:
Source: Option Adjusted Spreads (wallstreetmojo.com)
Example of Option Adjusted Spreads (OAS)
Using a Monte Carlo simulation model, ten volatility paths are derived, and each path has a weight of 10%. The cash flows on each path are discounted by short-term interest rates plus a spread on that path. The present value of each path is mentioned below:
Present Value if the Spread is
| Path | 70 bps | 75 bps | 80 bps | 85 bps |
|---|---|---|---|---|
| 1 | 92 | 90 | 85 | 83 |
| 2 | 74 | 71 | 67 | 65 |
| 3 | 68 | 64 | 61 | 59 |
| 4 | 77 | 75 | 70 | 68 |
| 5 | 96 | 90 | 86 | 83 |
| 6 | 74 | 71 | 67 | 65 |
| 7 | 86 | 84 | 81 | 79 |
| 8 | 90 | 87 | 84 | 80 |
| 9 | 80 | 78 | 75 | 72 |
| 10 | 85 | 82 | 78 | 75 |
If the market price of the security is $79.2, what is the option-adjusted spread?
If the market price of the security is $75, calculate the option-adjusted spread?
Solution
The theoretical value of the security is the weighted average of the present value of all the paths. Since each path carries the same weight hence taking the simple average would provide the same results.
Present Value if the Spread is
| Path | 70 bps | 75 bps | 80 bps | 85 bps |
|---|---|---|---|---|
| Sum of All (X) | 822 | 792 | 754 | 729 |
| No of paths (Y) | 10 | 10 | 10 | 10 |
| Average PV (X/Y) | 82.2 | 79.2 | 75.4 | 72.9 |
If the market price of the security is $ 79.2, then the corresponding OAS is 75 bps.
If the market price of the security is $ 75, then the option-adjusted spread is computed using linear interpolationInterpolationInterpolation is the mathematical procedure applied to derive value in between two points having a prescribed value. It approximates the value of a given function at a given set of discrete points. It can be applied in estimating varied concepts of cost, mathematics, statistics.read more.
Difference in bps (between 2 available PVs)
- = 75 – 80
- = -5 bps
The difference in PVs (between 2 available bps)
- = 75.4 – 72.9
- = $ 2.5
Additional OAS (base 80 bps)
- = -5 * (75.4-75) / 2.5
- = -0.8 bps
OAS Spread when the price is $ 75
- = 80 – (-0.8) bps
- = 80.8 bps
Important Points about Option Adjusted Spread
- The price of option-free bondsBondsA bond is financial instrument that denotes the debt owed by the issuer to the bondholder. Issuer is liable to pay the coupon (an interest) on the same. These are also negotiable and the interest can be paid monthly, quarterly, half-yearly or even annually whichever is agreed mutually.read more can be easily measured by discounting the cash flows using the benchmark yield curve. But this is not the case with the securities with embedded options. Volatility in the interest rates plays an important role in ascertaining whether the option is going to be invoked or not.
- The option-adjusted spread is a constant spread which is added to the prevailing interest rates to discount the cash flows. Such discounted cash flowsDiscounted Cash FlowsDiscounted cash flow analysis is a method of analyzing the present value of a company, investment, or cash flow by adjusting future cash flows to the time value of money. This analysis assesses the present fair value of assets, projects, or companies by taking into account many factors such as inflation, risk, and cost of capital, as well as analyzing the company's future performance.read more sum to the theoretical value of the security, which in turn indicates the market price of the security.
- OAS uses a number of scenarios carrying possibilities of numerous interest rate paths that are calibrated to the security yield curveYield CurveThe Yield Curve Slope is used to estimate the interest rates and changes in economic activities. It is a plot of bond yields of a particular issuer on the vertical axis (Y-axis) against various tenors/maturities on the horizontal axis (X-axis).read more. The cash flows are determined along all the paths, and the results are used in arriving at the price of the security.
- In the collateralized mortgage obligation (CMOCMOCollateralized Mortgage Obligations (CMOs) are a debt-security type that combines many mortgages & sell them as a single investment. Cash flow occurs when debtors repay the loans, following which the CMO investors get their returns. read more) market, the OAS on amortization class tranches goes along with the life of the tranches. OAS for shorter maturities is lesser, for medium-term notes are higher with the highest spread on longer-term notes. Hence, OAS turns out to be a bell-shaped curveBell-shaped CurveBell Curve graph portrays a normal distribution which is a type of continuous probability. It gets its name from the shape of the graph which resembles to a bell. read more.
- The difference between option-adjusted and zero-volatility spread provides the implied cost of embedded options in case of asset-backed securityAsset-backed SecurityAsset-backed Securities (ABS) is an umbrella term used to refer to a kind of security that derives its value from a pool of assets, such as bonds, home loans, car loans, or even credit card payments.read more.
- When talking about alternatives to OAS, binomial models and other fancier models can be used, but a lot of assumptions are required to be taken to determine the value using such models. Hence, the option-adjusted spread is preferred.
Advantages
- Helps in the computation of the price of a security with an embedded option.
- Reliable as the base calculation is similar to that of z-spread calculation.
- Prepayment probability is based on historical data rather than an estimation.
- Use of advanced models like Monte Carlo analysis in simulation.
Disadvantages
- Complex computation
- Difficult to implement
- Poor interpretation of OAS often results in a deformed view of the behavior of securities
- Prone to model risk
Limitations
Portfolio OAS is taken as the weighted average of the OAS of individual securities where weight is the market price of the securities. This limits the use of OAS to such users who want to inspect the daily contribution to return at present. But to extend its relevance to a wide array of users, the spreads should be weighted by both durations and market weights.
Conclusion
Despite involving complex calculations and placing reliance on sophisticated models, the option-adjusted spread has turned out to be an analytical tool for the evaluation of embedded securities. An improvisation in the areas of limitation can increase its popularity and usage manifold.
Recommended Articles
This has been a guide to Option Adjusted Spreads. Here we discuss the formula to calculate Option Adjusted Spreads (OAS) along with examples, advantages, and disadvantages. You can learn more about derivatives from the following articles –
50.3K subscribers