Net Present Value (NPV)

Net Present Value (NPV), most commonly used to estimate the profitability of a project, is calculated as the difference between the present value of cash inflows and the present value of cash outflows over the project's time period. If the difference is positive, it’s a profitable project and if it is negative, then it’s not worthy.

A net present value equation helps the user understand the time value of money. This data can be used to compare different projects and their profitability to decide which project is the most lucrative to accept. In corporate securities, NPV is often referred to as Discounted Cash Flow analysis. NPV can be calculated using tables, financial calculators, or spreadsheets.

Net Present Value (NPV) is a financial metric that assesses the profitability of an investment by comparing the present value of expected future cash flows to the initial investment. It considers the time value of money, recognizing that a dollar today is worth more than a dollar in the future.

A positive NPV indicates a potentially viable investment, as it suggests that the project will generate more value than its cost. A negative NPV suggests the opposite.

By discounting future cash flows, net present value analysis helps individuals and businesses make informed decisions about allocating resources and pursuing projects that enhance overall financial value.

The NPV formula has two parts-

• The first part talks about cash inflows from investments. When an investor looks at an investment, he is presented with the projected future values of the investments. He then can use the present value method to discount the future values and find out the cash inflows from the investments at the present date.
• The second part talks about the cost of investments in the project. It means how much an investor has to pay for the investments at the present date.

If the cost of investments is lesser than the cash inflows from the investments, then the project is quite good for the investor since he is getting more than what he is paying for. Otherwise, if the cost of investments is more than the cash inflows from the investments, then it’s better to drop the project since the investor has to pay more than what he is paying as of now.

Now that we have a basic understanding of the concept and its related factors, let us discuss the formula that shall act as a basis for our understanding of the intricacies of the concept.

NPV_{t=1 to T} = ∑X_t /

Here’s the Net Present Value formula (when cash arrivals are even):

NPV_{t=1 to T}  = ∑  X_t/(1 + R)^t – X_o

Where,

• X_t = total cash inflow for period t
• X_o = net initial investment expenditures
• R = discount rate, finally
• t = total time period count

The Net present value formula (when cash arrivals are uneven):

NPV = – X_o

Where,

• R is the specified return rate per period;
• C_i1 is the consolidated cash arrival during the first period;
• C_i2 is the consolidated cash arrival during the second period;
• C_i3 is the consolidated cash arrival during the third period, etc

Net present value analysis involves assessing the value of future cash flows from an investment or project in today's terms, accounting for the time value of money. Here's a step-by-step guide:

• Identify Cash Flows: Determine the expected cash flows generated by the investment over its lifespan. These can include initial investment costs, operating revenues, operating expenses, taxes, and salvage values.
• Select Discount Rate: Choose an appropriate discount rate that reflects the opportunity cost of capital or the required rate of return. This rate should consider the riskiness of the investment and the return expectations of investors.
• Discount Future Cash Flows: Apply the chosen discount rate to each future cash flow to calculate its present value.
• Calculate Initial Investment: Similarly, discount the initial investment cost back to its present value using the same formula.
• Sum Present Values: Add up all the present values calculated in step 3, including the present value of the initial investment. This gives you the total present value of expected cash flows.
• Calculate NPV: Subtract the total present value of cash flows from the present value of the initial investment. The formula is NPV = Total Present Value of Cash Flows - Present Value of Initial Investment.
• Interpret NPV: A positive NPV indicates that the investment is expected to generate more value than the initial cost, potentially making it a worthwhile venture. A negative NPV suggests that the investment may not be financially viable. The larger the positive NPV, the more attractive the investment.
• Decision: Use the calculated NPV as a decision-making tool. If NPV is positive, the project may be acceptable; if negative, it may not be a wise choice. Compare NPV results across different projects to determine the most beneficial option.

Now you can Master Financial Modeling with Wallstreetmojo's premium courses at special prices

Best Financial Modeling Courses by Wallstreetmojo

Now that we know the basics, formula and how to calculate using the net present value method, let us apply the knowledge to practical application through the examples below.

Example #1

Hills Ltd. would like to invest in a new project. The company has the following information for this new investment –

• Cost of the new investment as of now - $265,000
• The project will receive cash inflows as follows - 
  • Year 1 - $60,000
  • Year 2 - $70,000
  • Year 3 - $80,000
  • Year 4 - $90,000
  • Year 5 - $100,000

Find out the NPV and conclude whether this is a worthy investment for Hills Ltd. Assume the rate of return as 10%.

By using the above information, we can easily do the NPV Calculation of the new investment.

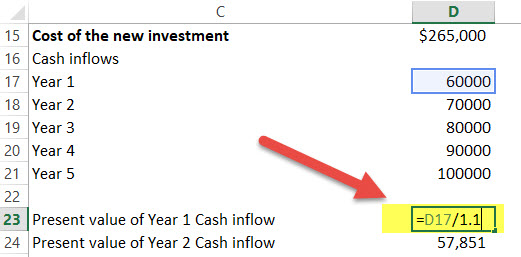
Cash Inflows from Investments = $60,000/1.1 + $70,000/1.1^2 + $80,000/1.1^3 + $90,000/1.1^4 + $100,000/1.1^5

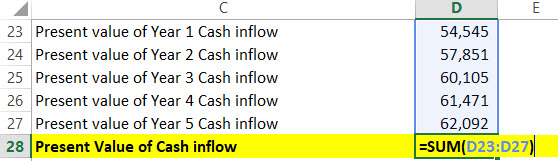
= 54,545.5 + 57,851.2 + 60,105.2 + 61,471.2 + 62,092.1 = 296,065.2

Net Present Value  = Cash Inflows from Investments – Cost of Investments

Or, Net Present Value  = $296,065.2 - $265,000 = $31,065.2

From the above result, we can be sure that this is a worthy investment; because the NPV of this new investment is positive.

Example #2

Alibaba will generate $1.2 billion of free cash flows in March’19. As we note below that Alibaba will generate a predictable positive Free Cash Flows.

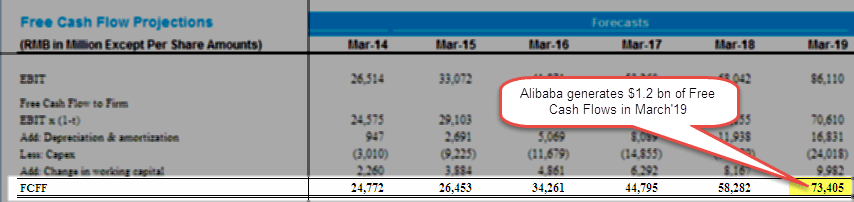
• Step 1 here is to apply the net present value formula to calculate the present value of the FCFF explicit period.
• Step 2 is to apply the net present value formula to calculate the PV of the terminal value.

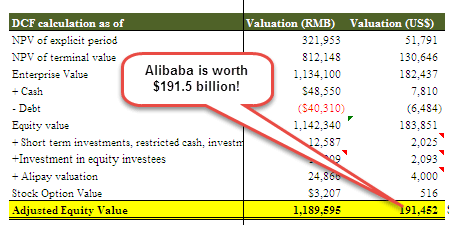
The sum total of the NPV Calculation in steps 1 and 2 gives us the total Enterprise Value of Alibaba.

Below is the table that summarizes Alibaba’s DCF Valuation output.

Advantages

By using this formula, the investors find out the difference between the cash inflows from the investments and the cost of investments, giving them a clear idea of the profitability of the investment. Let us understand the advantages of using the net present value method.

• It is very easy to calculate. Before making any decisions regarding investments, if the investor knows how to calculate NPV, they shall be able to make better decisions.
• Secondly, it compares the present value of both cash inflow and cash outflow. As a result, the comparison provides the right perspective for the investors to make the right decision.
• NPV offers the user a conclusive decision. After calculating this, they will directly get to know whether to go for the investments or not.
• It helps investors consider multiple investment options irrespective of heterogeneous initial costs, project durations, and cash flow patterns.
• It works in collaboration with metrics such as Internal Rate of Return (IRR) and payback method to provide a comprehensive and accurate financial analysis.  

Despite the advantages mentioned above, there are a few disadvantages of a net present value equation. Let us understand them through the discussion below.

• The accuracy of these calculations relies heavily on the precise estimation of future cash flows. If these estimates are unrealistic or inaccurate, the calculations could deliver misleading results.
• Inherently, it does not consider the scale of the investment. For instance, two different projects might have different initial investments but the NPV calculations could deliver similar cash flow requirements despite different risk profiles of these projects.
• Short-term projects might have higher NPV values, regardless of if long-term projects are able to generate more value over time.
• This concept conveniently ignores the capital constraints or limitations on available funds, which potentially leads to making average investment decisions.

You can use the following NPV Calculator

Let us now do the same example above in Excel.

This is very simple. You need to provide the two inputs of Cash Inflows from Investments and Cost of Investments.

You can easily calculate the NPV in the Excel template provided.

Step 1 - Find the present value of the cash inflows.

Step 2 - Find the sum total of the present values.

Step 3 - NPV Calculation = $296,065.2 - $265,000 = $31,065.2

This has been a guide to what is Net Present Value (NPV). Here we explain its formula, calculation, examples, advantages, disadvantages, and a calculator. You may also have a look at these articles below to learn more about Financial Analysis –

• Value Formula in Excel
• Discounted Payback Period Calculation
• NPV Function in Excel
• IRR vs NPV