Mean vs Median

Last Updated :

Blog Author :

Edited by :

Reviewed by :

Table Of Contents

Difference Between Mean and Median

Mean and median are two commonly used terms in mathematics. Mean is like an average of a given number. It sums up the numbers and divides them with the count of numbers which provides us with the mean. On the other hand, the median returns the middle number from the whole data set, if even. It adds the two middle numbers and divides them by 2, giving us the median.

Both measure central tendency and large data sets where analysis needs to be drawn and results are interpreted. Finally, mean, median, and mode are three measures of averages that show the dispersion of the data is from the mean or the average. These methods are used in statistics widely, whereas the mean value of the data is the most commonly used method among the three.

Difference Between Mean and Median

What is Mean?

Mean is a simple sum of all observations divided by the number of observations in an array. For example, if we talk about the average height or the mean height of a group of 5 people, we would calculate the mean by summing the height of 5 people divided by the number of people, 5.

Formula

Mean Formula = (Sum of all the observations/number of observations)

What is the Median?

The median is the middle number in the data array set, which separates the higher set of the data from the lower. Therefore, the data needs to be arranged in ascending order first to calculate the median of the data. Then, when the data set has cardinality, the mean of the middle two numbers in the data set needs to be taken. However, these two methods are often used interchangeably.

Formula

Median formula = (n+1)/2

when n is an odd number

Median = / 2

when n is an even number

Mean vs Median Infographics

Let us see the top differences between the mean and median.

Mean vs Median Key Differences

Mean is simple and can be applied to any data array set, whether even or odd. The median is slightly complex, and the data set needs to be arranged in ascending or descending order before calculation.
The mean is generally used for normal distributions, whereas the median is used for the skewed distribution data set.
The mean is simple, but it is not robust because it can contain outliers in the distributions and sometimes does not give the user the correct results for interpretation. On the other hand, the median method is robust and is better suited for skewed distributions to derive the central tendency of the date set and will give the user many accurate results when compared to mean.
There is only one formula for mean – the sum divided by the number of observations. At the same time, the median has two formulas. One odd where just the middle numbers from the dataset become the median. But, when we have an even data set, the middle of the two values are picked and divided by 2, giving us the median of the even data set.

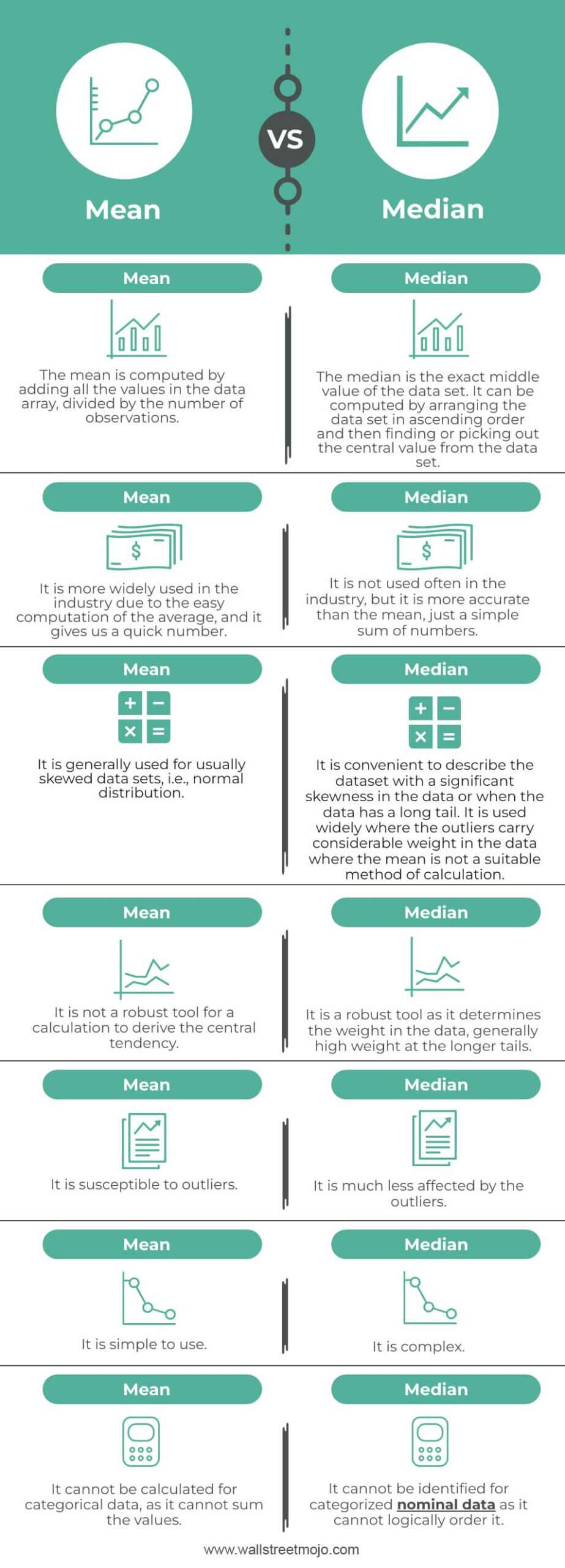
Mean vs Median Comparative Table

Mean	Median
The mean is computed by adding all the values in the data array, divided by the number of observations.	The median is the exact middle value of the data set. It can be computed by arranging the data set in ascending order and then finding or picking out the central value from the data set.
It is more widely used in the industry due to the easy computation of the average, and it gives us a quick number.	It is not used often in the industry, but it is more accurate than the mean, just a simple sum of numbers.
It is generally used for usually skewed data sets, i.e., normal distribution.	It is convenient to describe the dataset with a significant skewness in the data or when the data has a long tail. It is used widely where the outliers carry considerable weight in the data where the mean is not a suitable method of calculation.
It is not a robust tool for a calculation to derive the central tendency.	It is a robust tool as it determines the weight in the data, generally high weight at the longer tails.
It is susceptible to outliers.	It is much less affected by the outliers.
It is simple to use.	It is complex.
It cannot be calculated for categorical data, as it cannot sum the values.	It cannot be identified for categorized nominal data as it cannot logically order it.

Conclusion

Apart from the mean and median, one more method is often used to measure central tendency: the mode. A mode is a value that most frequently occurs in the data set. It has an advantage over the mean and median and can be found for numerical and categorized data sets.

Despite the existence of mode and median and their superiority of better results and analysis over the mean, the mean is still the most appropriate measure of central tendency, especially if the data set is a normal distribution and the data is usually skewed.

As a good analyst, the central tendency should be measured with all three data methods. Then, the variance in the analysis should be pondered and carefully analyzed to produce better and more accurate results in the data set.