What is Marginal Benefit?
Marginal Benefit helps an organization to determine the optimal level of benefit derived from consumption and calculates the estimated quantity of its product/ service which will be demanded by the market, thereby, increasing cost efficiency in running a business. In short, it helps an organization to run its business more efficiently.
Marginal benefit is the progressive increase in favor of a consumer as a result of increased consumption by an extra unit of product or service purchased. The consumer’s satisfaction tends to decrease as consumption increases.
Marginal Benefit Formula
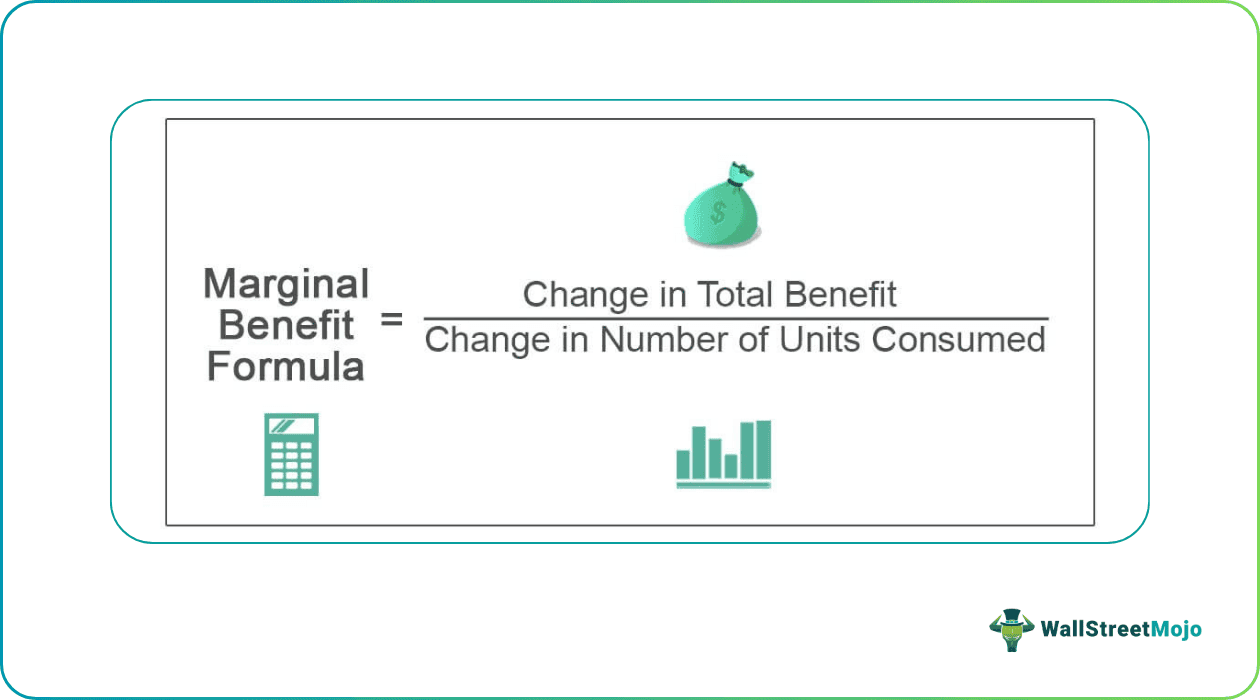
Marginal Benefit Formula = Change in Total Benefit / Change in Number of Units Consumed
You are free to use this image on your website, templates etc, Please provide us with an attribution linkHow to Provide Attribution?Article Link to be Hyperlinked
For eg:
Source: Marginal Benefit (wallstreetmojo.com)
Change in Total Benefits
This part comprises the change in total benefit and is derived by deducting the overall benefit of the current consumption from previous consumption. Let us develop a better understanding with the help of the following example. Say by consuming first banana, a consumer gains benefit of 10 units, whereas the second banana leads to the total benefit of 18. To arrive at the change in total Benefit between the second and first banana, we need to deduct the total Benefit of the first banana from the second banana. The result is a total Benefit of 8 (18 – 10).
Change in Number of Units Consumed
This part comprises calculating the change in the number of units consumed. It is derived by deducting the amount of the unit that is currently being consumed from a previously consumed unit. The change in units consumed from the second and first banana is 1 (2 – 1).
When both parts are calculated, the marginal benefit is derived by dividing the change in total Benefit by the difference in the number of units consumed.
Examples
Example #1
Suppose a consumer Harry buys and consumes an ice cream, let the benefit derived from the ice cream is measured as 50 units. Harry consumes another three ice cream. The benefit derived from 2nd, 3rd, and 4th ice cream is 40, 35, and 25. Calculate marginal benefit for 1st & 2nd and 1st & 3rd unit of Ice cream.
Solution:
Use the given data for the calculation
The calculation for 1st and 2nd Ice Cream can be done as follows:
1st and 2nd ice cream is (50-40) / (2nd – 1st unit)
Marginal Benefit for 1st and 2nd Ice Cream = 10
The calculation for 3rd and 1st Ice Cream can be done as follows:
Benefit for 3rd and 1st ice cream is (50 – 35) / (3rd – 1st unit)
Benefit for 3rd and 1st Ice Cream will be –
Marginal Benefit for 3rd and 1st Ice Cream = 7.5
Example #2
Mr. Peter runs a business of selling tea. Based on past selling experience, he has estimated benefit derived from consuming his tea mentioned as follows:
| Quantity of Tea | Total Utility |
|---|---|
| 1 | 300 |
| 2 | 540 |
| 3 | 670 |
| 4 | 780 |
| 5 | 880 |
You are required to calculate marginal benefit for each extra unit sold.
Solution:
Marginal Benefit for Quantity of Tea One = (300-0)/(1-0)
Similarly, we can calculate the marginal benefit for the remaining quantity of tea.
Example #3
Let’s say Mr. Harry sells ice cream at $10 each. The variable cost of making is $5 per unit. This leaves a gross profit of $5 per unit. (Fixed cost ignored for simplicity).
Solution:
- Current Gross Profit: 720
- Previous Gross Profit: 500
- Current Sales Volume: 180
- Previous Sales Volume: 100
On a Sunday, he sells 100 units leading to a gross profitGross ProfitGross Profit shows the earnings of the business entity from its core business activity i.e. the profit of the company that is arrived after deducting all the direct expenses like raw material cost, labor cost, etc. from the direct income generated from the sale of its goods and services.read more of $5 x 100 units or $500.
But for increasing sales, harry decides to lower the price to $9 each. At this price, you would make a gross profit of $4 per unit.
Due to the reduced prices, sales volume increases to 180 units. The first 100 consumers agreed to pay $10, so they’re even happier to pay $9. Further, 75 more customers joined and are willing to pay $9. The gross profit is now 720.
The calculation can be done as follows:
The marginal benefit will be ($720-$500)/(180 units – 100 units)
The final sales price may be calculated by the seller based on different factors affecting its business.
Relevance and Uses
- Based on the optimal level of benefit, an organization may prepare the budget for quantity to be produced.
Key Takeaways
- The change in the number of Benefits derived by the customer by increasing consumption by one additional unit of goods/ service is a marginal benefit.
- It is inversely related to consumption, i.e., with the increase in consumption, marginal benefit decreases.
- When the production or service increases, the change in cost that incurs is the marginal cost of production.
- It helps in determining the most efficient level of service or product demanded.
- Also, it helps to achieve economies of scale.
Recommended Articles
This article has been a guide to Marginal Benefit and its definition. Here we discuss how to calculate marginal benefit using its formula along with practical examples and downloadable excel template. You can learn more about financial analysis from the following articles –
58.6K subscribers