Interest on Loan

Interest on loan refers to the amount that a borrower is obligated to pay or a depositor is supposed to earn on a principal sum at a pre-determined rate, which is known as the rate of interest and the formula for interest can be derived by multiplying the rate of interest, the outstanding principal sum and the tenure of the loan or deposit.

The calculation of interest is an important concept to understand because it is an indispensable part of the income statement of any company. Interest on loan accounting can either impact the income side in the form of interest earned on an investment or affect the cost in the form of interest expense charged on the debt.

Interest on loan refers to the cost of borrowing. It is a fundamental concept in finance and is applicable to loans such as educational, personal, and business to remunerate the lender for the risk they take and opportunity costs related to providing the borrower with funds.

Typically, bank interest on loan is expressed as a percentage of the principal amount that the borrower has been provided with, and this percentage is charged over a certain period. The ‘principal’ here refers to the initial amount borrowed. The interest rate is decided based on multiple factors, such as the borrower’s creditworthiness, the type of loan, and the prevailing rates in the market.

For lenders, this incentive allows them to extend a line of credit to their clients and are compensated not just for the risks taken by them but also for the opportunity cost of lending. Moreover, it is often found that both private and formal lenders charge higher interest rates for borrowers with average or poor credit history as the risk of default or complete non-repayment is significantly higher.

For borrowers, understanding the intricate details of the loan along with the interest rate, tenure, repayment schedule, and compounding frequency, if applicable, can help them compare rates from different banks or credit-extending entities and choose the loan that best fits their circumstances.

It is also important to understand that there are two major types of interest- simple interest and compound interest, about which we shall learn in detail in this article. Until then, let us remember that it is important for both the lender and the borrower to understand even the minute details of the loan agreement to ensure they are agreeing with full knowledge.

Let us understand the formula that helps borrows figure out the lowest interest on loan from different lenders:

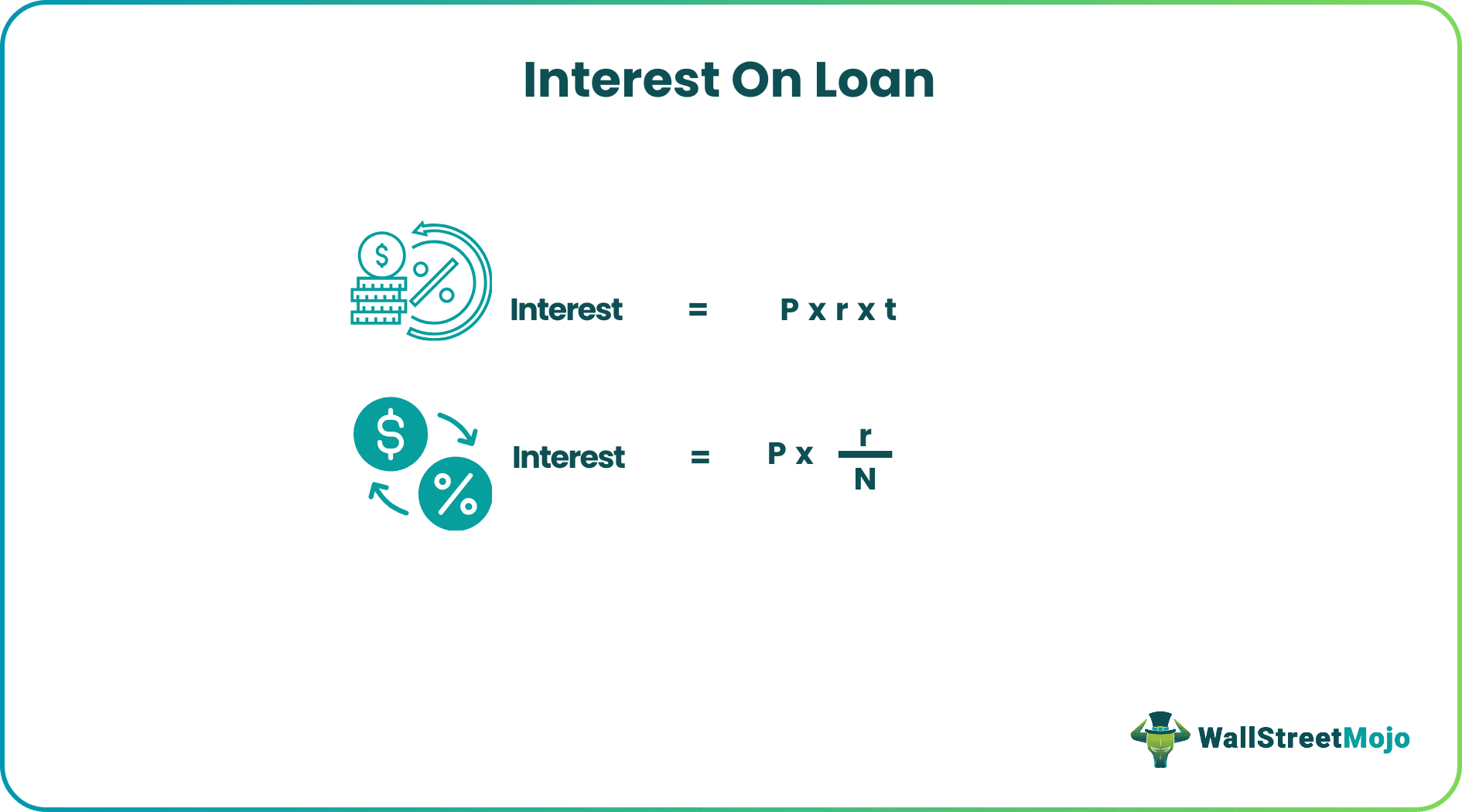
Interest on Loan = P * r * t

where,

• P = Outstanding principal sum
• r = Rate of interest
• t = Tenure of loan / deposit

In the case of periodic interest payment (such as monthly, quarterly, etc.), the equation for interest payment can be derived by multiplying the rate of interest and the outstanding principal sum and then dividing the result by the number of periodic payments during the year.

Mathematically, Interest Payment is represented as,

Interest on Loan = P * r / N

where,

• P = Outstanding principal sum
• r = Rate of interest
• N = number of periodic payment per year

To find out the lowest interest on loan from any lender, the step-by-step guide below can be followed:

1. Firstly, figure out the outstanding principal sum of the loan or deposit, and it is denoted by ‘P.’ Please keep in mind that the outstanding principal is the balance at the beginning of the year.

    

2. Next, figure out the rate of the interest rate for the given loan or deposit, which is denoted by ‘r.’
    

    

3. Next, figure out the tenure of the loan or deposit, and it is denoted by ‘t.’ The tenure of the facility is the number of years remaining until its maturity.

    

4. Finally, the interest can be derived by multiplying the outstanding principal sum (step 1), the rate of interest (step 2), and the tenure of the loan or deposit (step 3) as shown below,

    

Interest = P * r * t

Let us understand the different types of interest on loan account metric through the detailed discussion below.

1. Simple Interest: It is calculated based on the initial amount or principal amount throughout the loan term. Therefore, the interest amount remains the same for each period, thereby, there is a linear growth of the total interest paid throughout the tenure of the loan.
2. Compound Interest: This form of calculating interest takes both the principal amount and the interest accumulated from the previous periods as well. The interest is then calculated on the total amount, leading to exponential growth in the total interest paid throughout the tenure.
3. Fixed Interest Rate: Under this form of interest, the interest rate remains constant through the term of the loan. This helps borrowers take advantage of the predictability of their monthly payments and ease their financial budgeting and planning.
4. Variable Interest Rate: This interest rate can fluctuate based on economic indicators or market conditions. Borrowers may end up paying different amounts towards their EMI on a monthly basis as there is an element of uncertainty.
5. Nominal Interest Rate: It is the disclosed or stated rate of interest before taking compounding or inflation into consideration. It is used for straightforward calculations. However, the numbers might not reflect the actual cost of borrowing.
6. Effective Interest Rate: It reflects the true cost of borrowing by adding compounding and any additional fees into the calculation. It also provides an accurate idea of the financial impact the loan might have on the borrower.

Now that we understand the formula, how to calculate, and the types of interest on loan accounting, let us understand its practicality through the examples below.

Example #1

Let us take an example, Trevor, who has deposited his money at ABC Bank Ltd. As per the bank policy, Trevor has been offered an interest rate of 6% on a sum of $1,000 that has been deposited for a period of 3 years. Calculate the interest to be earned by Trevor at the end of 3 years.

Solution:

Given,

• Outstanding principal sum, P = $1,000
• Rate of interest, r = 6%
• Tenure of deposit, t = 3 years

Use the above data for the calculation of interest.

The interest earned by Trevor can be calculated as,

Interest = $1,000 * 6% * 3

Interest will be -

Interest = $180

Therefore, Trevor will earn an interest of $180 at the end of 3 years.

Example #2

Let us take another example of Smith, who has borrowed a sum of $5,000 from XYZ Bank Ltd for a period of 3 years. The rate of interest charged for the facility is 8%, and the loan has to be repaid in 6 equal half-yearly payments of $954. Calculate the interest on a loan to be paid by Smith at the end of 1^st year, 2^nd year, and 3^rd year.

Solution:

Given,

• Outstanding principal sum, P = $5,000
• Rate of interest, r = 8%
• Number of payments per year, N = 2 (since half-yearly payments)

Use the above data for calculation of interest charged for 1st six months.

So, calculation of interest charged for 1st six months can be done as follows -

Interest Charged for 1st Six Months = $5,000 * 8% / 2

Interest Charged for 1st Six Months will be -

Interest Charged for 1st Six Months = $200

Calculation of Outstanding Principal Sum after 1^st Six Months will be -

Outstanding Principal Sum after 1^st Six Months will be -

Outstanding principal sum after 1^st six months = $5,000 - ($954 - $200)

Outstanding Principal Sum after 1^st Six Months = $4,246

Similarly, we can do the calculation of outstanding principal and interest charged for months 1 to 5.

Let us understand the tax treatment for private or bank interest on loan based on the nature of loans through the detailed explanation below.

• Mortgage: Interest paid towards mortgage loans are often tax-deductible. The deduction is applicable for both primary and secondary residences as well but are subject to specific limits. The Tax Cuts and Jobs Act of 2017 made changes to these capping the deductible mortgage interest on new loans.
• Student Loans: Eligible student loans are often eligible for deductions but to a specific limit. Borrowers meeting the income criteria can claim this deduction while filing their income taxes.
• Business Loans: Entrepreneurs can deduct interest paid on loans used for genuine business purposes.