Implied Volatility Formula

Publication Date :

30 Oct, 2019

Blog Author :

Edited by :

Reviewed by :

Table Of Contents

Formula to Calculate Implied Volatility Formula?

Implied volatility is one of the important parameters and a vital component of the Black-Scholes model, an option pricing model that shall give the option's market price or market value. Implied volatility formula shall depict where the volatility of the underlying in question should be in the future and how the marketplace sees them.

When one does reverse engineering in the black and Scholes formula, not to calculate the value of option value, but one takes input such as the option's market price, which shall be the intrinsic value of the opportunity. Then one has to work backward and then calculate the volatility. The volatility implied in the price of the option is thus called implied volatility.

C = SN (d₁) - N (d₂) Ke ^-rt

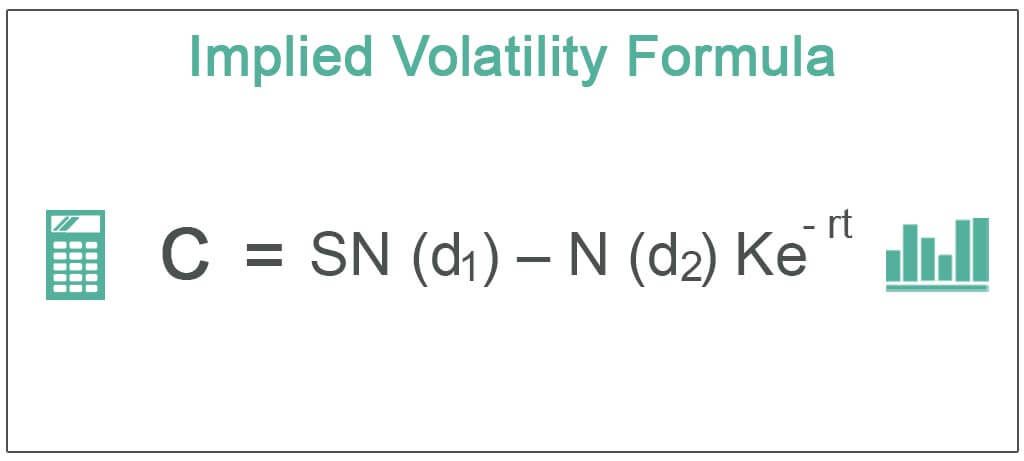
Implied Volatility Formula — *You are free to use this image on your website, templates, etc..* *Please provide us with an attribution link*

Where,

C is the Option Premium
S is the price of the stock
K is the Strike Price
r is the risk-free rate
t is the time to maturity
e is the exponential term

NOTE:
One has to work backward in the above formula to calculate implied volatility.

Key Takeaways

Implied volatility is essential in the Black-Scholes option pricing model. It predicts future volatility of the underlying asset and reflects the market's perception of it.
One will better assess public perceptions of market or stock volatility with forward-looking implied volatility. It is possible to compare the implied volatility to historical volatility and draw conclusions based on such examples.
It should be emphasized that implied volatility cannot predict an option's direction. It may represent the level of risk the trader is taking.

Calculation of the Implied Volatility (Step by Step)

The calculation of implied volatility can be done in the following steps:

Gathered the inputs of the Black and Scholes model, such as the Market Price of the underlying, which could be stock, the market price of the option, the strike price of the underlying, the time to expire, and the risk-free rate.
One must input the above data in the Black and Scholes Model.
Once the above steps are completed, one needs to start doing an iterative search by trial and error.
One can also do interpolation, which could be near the implied volatility, and by doing this, one can get an approximate nearby implied volatility.
This is not simple to calculate as it requires care at every stage to compute the same.

Examples

Example #1

Assume that the money call price is 3.23, the market price of the underlying is 83.11, and the strike price of the underlying is 80. There is only one day left for the expiration, assuming the risk-free rate is 0.25%. You are required to calculate the implied volatility based on the given information.

Solution

We can use the below Black and Scholes formula to calculate approximate Implied Volatility.

Use the below-given data for the calculation of implied volatility.

Call Option Value: 3.23
Stock Price: 83.11
Strike Price: 80.00
Risk Free Rate:0.25%

C= SN (d₁) - N (d₂) Ke ^-rt

3.23 = 83.11 x N(d1) – N(d2) x 80 x e^-0.25%*1

Using iterative and trial and error methods, we can try calculating at Implied Volatility, say at 0.3, where the value shall be 3.113, and at 0.60, the value shall be 3.24. Hence the Vol lies between 30% and 60%.

Trial and Error Method – Call Price at 30%

=$83.11*e^{(-0.00%*0.0027)})*0.99260-$80.00*e^{(-0.25%*0.0027)}*0.99227

=$3.11374

Trial and Error Method - Call Price at 60%

=$83.11*e^{(-0.00%*0.0027)})*0.89071-$80.00*e^{(-0.25%*0.0027)}*0.88472
=$3.24995

Now we can use the interpolation method to calculate the implied volatility at which it shall exist:

= 30% + (3.23 – 3.11374)/ (3.24995 – 3.11374) x (60% - 30%)
=55.61%

Therefore, the implied Vol shall be 55.61%.

Example #2

Stock XYZ has been trading at $119. Mr. A has purchased the call option at $3, which has 12 days remaining to expire. The choice had a strike price of $117, and you can assume the risk-free rate at 0.50%. Mr. A, a trader, wants to compute the implied volatility based on the above information.

Solution

We can use the Black and Scholes formula below to compute approximate Implied Volatility.

Use the below-given data for the calculation of implied volatility.

Call Option Value: 3.00
Stock Price: 119.00
Strike Price: 117.00
Risk Free Rate: 0.50%
Time to Expire: 12.00

C= SN (d₁) - N (d₂) Ke ^-rt

3.00 = 119 x N(d1) – N(d2) x 117 x e^{-0.25%*12/365}

Using iterative and trial and error methods, we can try calculating at Implied Volatility, say at 0.21, where the value shall be 2.97, and at 0.22, the value shall be 3.05; hence the vol lies between 21% and 22%.

Trial and Error Method – Call Price at 21%

=$119.00*e^{(-0.00%*0.0329)})*0.68028-$117*e^{(-0.50%*0.0329)}*0.66655
=$2.97986

Trial and Error Method - Call price at 22%

=$119.00*e^{(-0.00%*0.0329)})*0.67327-$117*e^{(-0.50%*0.0329)}*0.65876
=$3.05734

Now we can use the interpolation method to calculate the implied volatility at which it shall exist:

= 21% + (3. – 2.97986) /(3.05734 – 2.97986)x (22% - 21%)
=21.260%

Therefore, the implied Vol shall be 21.26%

Example #3

Assuming the stock price of Kindle is $450, its call option is available at $45 for the strike price of $410 with a risk-free rate of 2%, and there are three months to the expiry for the same. Based on the above information, you are required to compute implied volatility.

Solution:

We can use the Black and Scholes formula below to compute approximate Implied Volatility.

Use the below-given data for the calculation of implied volatility.

Call Option Value: 45.00
Stock Price: 450.00
Strike Price: 410.00
Risk Free Rate: 2.00%
Time to Expire: 90.00

C= SN (d₁) - N (d₂) Ke ^-rt

45.00 = 450 x N(d1) – N(d2) x 410 x e^{-2.00% *(2*30/365)}

Using iterative and trial and error methods, we can try calculating at Implied Volatility, say at 0.18, where the value shall be 44.66, and at 0.19, the value shall be 45.14; hence the vol lies between 18% and 19%.

Trial and Error Method – Call Price at 18%

=$450.00*e^{(-0.00%*0.2466)})*0.87314-$410*e^{(-2.00%*0.2466)}*0.85360
=$44.66054

Trial and Error Method - Call Price at 19%

=$450.00*e^{(-0.00%*0.2466)})*0.86129-$410*e^{(-2.00%*0.2466)}*0.83935
=$45.14028

Now we can use interpolation method to calculate the implied volatility at which it shall exist:

= 18.00% + (45.00 – 44.66054) / (45.14028– 44.66054) x (19% - 18%)
=18.7076

Therefore, the implied Vol shall be 18.7076%.

Refer to the above given excel sheet for detailed calculations.

Relevance and Uses

Being forward-looking implied volatility will aid one in gauging the sentiment about the volatility of the market or a stock. This implied volatility can be compared with historical volatility, and hence decisions can be made based on those cases. However, it has to be noted that the implied volatility will not forecast in which direction an option is leaning. This could be the measure of risk that the trader is putting into.