Definition of Extrapolation Formula

Extrapolation Formula refers to the formula that is used in order to estimate the value of the dependent variable with respect to an independent variable that shall lie in range which is outside of given data set which is certainly known and for calculation of linear exploration using two endpoints (x1, y1) and the (x2, y2) in the linear graph when the value of point which has to be extrapolated is “x”, a formula that can be used is represented as y1+ [(x−x₁) / (x₂−x₁)] *(y₂−y₁).

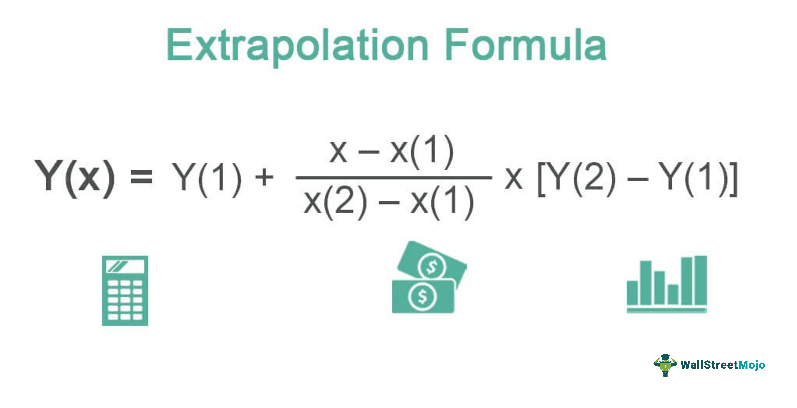
Y(x) = Y(1)+ (x- x(1)/x(2)-x(1)) * (Y(2) – Y(1))

You are free to use this image on your website, templates etc, Please provide us with an attribution linkHow to Provide Attribution?Article Link to be Hyperlinked
For eg:
Source: Extrapolation Formula (wallstreetmojo.com)

Calculation of Linear Extrapolation (Step by Step)

The formula for Linear Extrapolation can be divided into the following steps:

The data first needs to be analyzed whether the data is following the trend and whether the same can be forecasted.
There should be two variables where one has to be a dependent variable, and the second has to be an independent variable.
The numerator of the formula starts with the previous value of a dependent variable, and then one needs to add back the fraction of the independent variableIndependent Variable Independent variable is an object or a time period or a input value, changes to which are used to assess the impact on an output value (i.e. the end objective) that is measured in mathematical or statistical or financial modeling.read more as one does while calculating for mean for class intervals.
Finally, multiply the value arrived in step 3 by a difference of immediate given dependent values. After adding step 4 to the value of the dependent variable will yield us the extrapolated value.

Examples

You can download this Extrapolation Formula Excel Template here – Extrapolation Formula Excel Template

Example #1

Suppose that the value of the certain variables is given below in the form of (X, Y):

(4, 5)
(5, 6)

Based on the above information, you are required to find the value of Y(6) using the extrapolation method.

Solution

Use the below-given data for calculation.

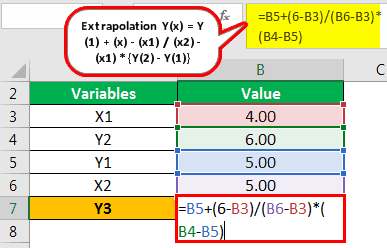
X1: 4.00
Y2: 6.00
Y1: 5.00
X2: 5.00

Calculation of Y(6) using extrapolation formula is as follows,

Extrapolation Y(x) = Y(1) + (x) – (x1) / (x2) – (x1) x {Y(2) – Y(1)}

Y(6) = 5 + 6 – 4 / 5 – 4 x (6 – 5)

The answer will be –

Y3 = 7

Hence, the value for Y when the value of X is 6 will be 7.

Example #2

Mr. M and Mr. N are students of the 5^th standard, and they are currently analyzing the data given to them by their math teacher. The teacher has asked them to compute the weight of students whose height will be 5.90 and has informed that the below set of data follows linear extrapolation.

X	Height	Y	Weight
X1	5.00	Y1	50
X2	5.10	Y2	52
X3	5.20	Y3	53
X4	5.30	Y4	55
X5	5.40	Y5	56
X6	5.50	Y6	57
X7	5.60	Y7	58
X8	5.70	Y8	59
X9	5.80	Y9	62

Assuming that this data follows a linear series, you are required to calculate the weight, which would be dependent variable Y in this example when the independent variable x (height) is 5.90.

Solution

In this example, we now need to find out the value, or in other words, we need to forecast the value of students whose height is 5.90 based on the trend given in the example. We can use below extrapolation formula in excel to calculate the weight, which is a dependent variable for a given height, which is an independent variable

Calculation of Y(5.90) is as follows,

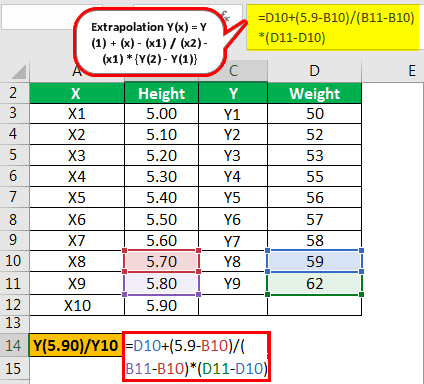
Extrapolation Y(5.90) = Y(8) + (x) – (x8) /(x9) – (x8) x [Y(9) – Y(8)]
Y(5.90) = 59 + 5.90 – 5.70 / 5.80 – 5.70 x (62 – 59)

The answer will be –

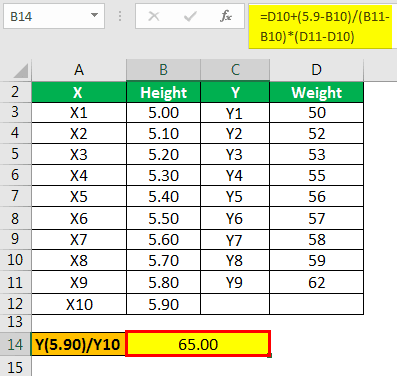
= 65

Hence, the value for Y when the value of X is 5.90 will be 65.

Example #3

Mr. W is the executive director of the company ABC. He was concerned with the sales of the company being following a downward trend. He has asked his research department to produce a new product which shall follow increasing demand as and when the production increases. After 2 years, they develop a product which faced increasing demand.

Below are the details of the last few months:

X (Production)	Produced (Units)	Y (Demand)	Demanded (Units)
X1	10.0	Y1	20.00
X2	20.00	Y2	30.00
X3	30.00	Y3	40.00
X4	40.00	Y4	50.00
X5	50.00	Y5	60.00
X6	60.00	Y6	70.00
X7	70.00	Y7	80.00
X8	80.00	Y8	90.00
X9	90.00	Y9	100.00

They observed that since this was a new product and cheap product and hence initially, this would follow linear demand until a certain point.

Hence moving forward, they would first forecast the demand and then compare them with actual and produce accordingly as this has demanded huge cost for them.

The marketing manager wants to know what the units would be demanded if they produce 100 units. Based on the above information, you are required to calculate the demand in units when they produce 100 units.

Solution

We can use the below formula to calculate the demands in units, which is the dependent variable for given units produce, which is an independent variable.

Calculation of Y(100) is as follows,

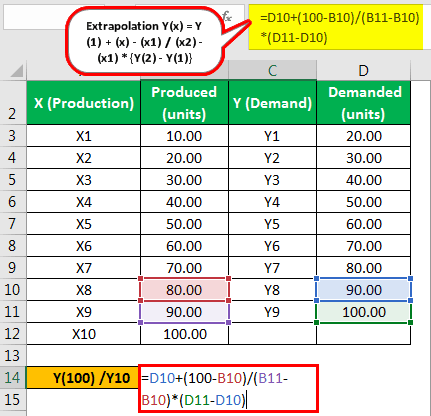
Extrapolation Y(100) = Y(8) + (x)- (x8) / (x9) – (x8) x [ Y(9) – Y(8)]
Y(100) = 90 + 100 – 80 / 90 – 80 x (100 – 90)

The answer will be –

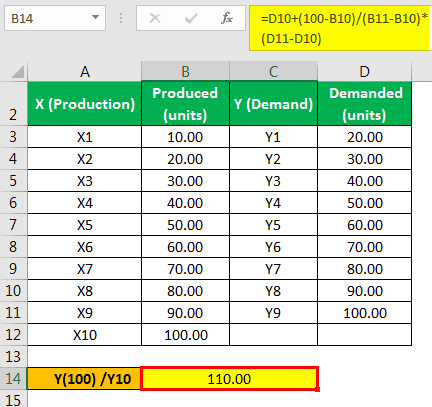
= 110

Hence, the value for Y when the value of X is 100 will be 110.

Relevance and Uses

It is mostly used to forecast the data which is out of the current range of the data. In this case, one is assuming that the trend shall continue for given data and even outside that range, which shall not be the case always, and hence extrapolation should be used very cautiously, and instead, there is a better method to do the same is the use of interpolation method.

19th/20th September 2020	Saturday - Sunday	9 am IST to 5 pm IST
26th/27th September 2020	Saturday - Sunday	9 am IST to 5 pm IST

Extrapolation Formula

Definition of Extrapolation Formula