What is the Effective Yield?
Effective yield can be defined as a yearly rate of return at a periodic rate of interest and the method is proclaimed to be one of the effective measures of an equity holders’ return as it takes compounding into its due consideration unlike nominal yield method and it is also based on an assumption that an equity holder is eligible for reinvesting his or her coupon payments at a coupon rate.
Table of contents
Explanation
It is also better known as annual percentage yield (APY). It is way different from periodic yield, and the two must not be confused with one another. Periodic yield can be defined as the yield pertaining to any period, which could either be on a monthly, half-yearly, or quarterly basis, whereas it can be defined as the annual returnThe Annual ReturnThe annual return is the income generated on an investment during a year as a percentage of the capital invested and is calculated using the geometric average. This return provides details about the compounded return earned yearly and compares the returns supplied by various investments like stocks, bonds, derivatives, mutual funds, etc.read more or yield. It takes compoundingCompoundingCompounding is a method of investing in which the income generated by an investment is reinvested, and the new principal amount is increased by the amount of income reinvested. Depending on the time period of deposit, interest is added to the principal amount.read more into consideration and assumes that the coupon payments are already reinvested. This method is of great use for making a comparison of assets that pay at least twice in a year.
Effective Yield Formula
The formula is provided below:
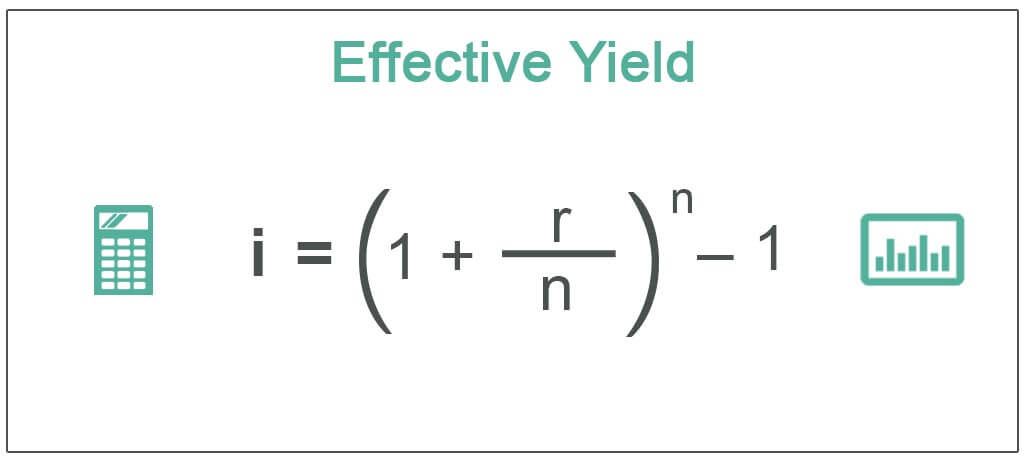
Effective Yield Formula = [1 + (r/n)]n – 1
Here, ‘r’ represents a nominal rate, and ‘n’ represents no. of payments received annually.
You are free to use this image o your website, templates, etc, Please provide us with an attribution linkHow to Provide Attribution?Article Link to be Hyperlinked
For eg:
Source: Effective Yield (wallstreetmojo.com)
How to Calculate Effective Yield?
It can be calculated by following the steps provided and discussed below:
Step #1 – In the first step, the users must determine “n” or a number of payments that are received during the year. Securities that pay twice in a year or, in other words, pay every 6 months, and then for such financial securities, the ‘n’ shall be 2. Similarly, financial securities that pay every quarterly and monthly will have a number of periods as 4 and 12, respectively.
Step #2 – In the next step, the users will need to determine ‘i’ that is the rate of interest (ROI). This rate of interest is already mentioned in financial security.
Step #3 – In the third step, the users will be required to divide the rate of interest and that too in decimal form by the number of payment intervals determined in Step 1.
Step #4 – In the fourth step, the users will need to sum up 1 + (i/n).
Step #5 – In the fifth step, the users will need to take the value derived in Step 4 and determine exponent ‘n.’
Step #6 – In the sixth step, which is also the last step, the users will be required to deduct 1 for the annualized yield.
Examples of Effective Yield
Example #1
Purchases the bond of Company ABC that has a 6 %coupon. The nominal rate is 6%. Calculate the effective yield if the interest is paid annually.
Solution
Given,
- r = 6 %
- n= 1
- i = ??
If the interest paid is annually, then the number of payment periods in a year is 1.
The calculation for the determination of A’s yield on her 6% coupon bondCoupon BondCoupon bonds pay fixed interest at a predetermined frequency from the bond’s issue date to the bond’s maturity or transfer date. The holder of a coupon bond receives a periodic payment of the stipulated fixed interest rate.read more is as follows:
- = (1+(6%/1))^1-1
- i = 6 %
Example #2
B purchases the bond of Company XYZ that has a 5% coupon. If the interest is semi-annually paid then what would be the B’s effective yield on her 5% coupon bond?
Solution
Given,
- r = 5 %
- n= 2
- i = ??
If the interest is half-yearly paid, then the number of payment periods in a year is 2. The nominal rate is 5 percent.
Therefore, the calculation for the determination of B’s yield on her 5 percent coupon bond is as follows-
- =(1+(5%/2))^2-1
- i = 5.062%
Example #3
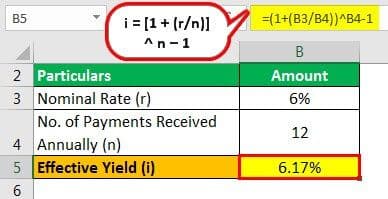
C purchases the bond of Company ABC that has a 6% coupon. If the interest is paid every month, then determine what would be the C’s effective yield on her 6% coupon bond?
Solution
Given,
- r = 6 %
- n= 12
- i = ??
If the interest is being paid every month, then the number of payment periods in a year is 12. The nominal rate is 6 percent.
Therefore, the calculation for the determination of C’s yield on her 6 percent coupon bond is as follows:
- =(1+(6%/12))^12-1)
- i = 6.17%
Conclusion
Effective yield is also termed as annual percentage yield or APY and is the return generated for every year. Its formula is i = [1 + (r/n)]n – 1.
This method is highly preferred by most of the investors since the method, unlike all other methods, takes compounding into its due consideration and also assumes that the investors are eligible for reinvesting their coupon payments at the coupon ratesCoupon RatesThe coupon rate is the ROI (rate of interest) paid on the bond's face value by the bond's issuers. It determines the repayment amount made by GIS (guaranteed income security). Coupon Rate = Annualized Interest Payment / Par Value of Bond * 100%read more.
This method is way different from the nominal method, and hence, the two must not be confused with one another. If the payments received from the bonds are invested again, then the effective yield of an investor shall be higher than the nominal yield or mentioned coupon yield as a result of compounding.
It has few drawbacks, too, since it is based on the assumption that the coupon payments are required to be invested back into another cycle that pays the same rate of interest. However, this may not always be possible merely because of the fact that the rate of interest is bound to fluctuate periodically as a result of various prevalent factors in an economy.
Recommended Articles
This has been a guide to what is Effective Yield?. Here we discuss steps to calculate effective yield along with its formula and examples. You can learn more about from the following articles –
Leave a Reply