Table Of Contents
What Is Effective Interest Rate?
Effective Interest Rate (EIR) is the rate on which the actual return on investment and rate of interest on a loan is calculated, considering compounding over a period. It offers the exact estimate of the amount the investors are likely to receive on their investment, and the borrowers are liable to pay as interest.

Also known as the Effective Annual Rate (EAR), it indicates the percentage adjusted with respect to the compound interest calculated over a period. Deriving this interest rate helps assess the real cost of borrowing and the return on investment that one is subject to come across.
Table of contents
- Effective Interest Rate, also known as annual equivalent rate, is the rate of interest on the financial instrument that is actually paid or earned by the person based on the effect of the compounding over the period.
- Compounding changes the interest rate. This is why the interest rate written on the instrument is not an EIR or annual equivalent rate for the investor.
- Even if the difference between the effective and nominal interest rates is meager, they will still significantly affect the actual return or percentage of interest at the end.
- The amount derived after calculation indicates the cost of borrowing or the returns on investment involved.
Effective Interest Rate Method Explained
The effective interest rate gives an overall idea of the true returns and interest payments that people in concern need to know. The true yield and cost of borrowing can be found when the compounding period for the loans is known. Compounding changes the interest rate. That’s why the interest rate written on the instrument is not an effective interest for the investor or lender/borrower.
Though the EIR and the annual rate are considered to be always the same, they still differ. This is because the interest gets compounded a number of times every year. It could be done annually, semi-annually, quarterly, or monthly. This, thereby, affects the annual equivalent rate, making it different from the nominal interest rate.
The EIR helps investors find better investment opportunities with higher actual returns. Based on the results obtained, they can decide which investment vehicle to spend on. Similarly, the EAR also lets the borrowers identify the lenders offering loans at a lower interest rate when compounded over a period. In short, these rates help assess and invest or borrow based on convenience and suitability.
Formula
The effective interest rate formula is:
EIR = (1 + i/n)n – 1
Here,
- i = Annual interest rate mentioned in the instrument
- n = Number of compounding periods per year
Examples
Let us consider the following examples to understand the effective interest rate meaning even better:
Example #1 - (Excel Function)
For finding the EIR or Annual Equivalent Rate in excel, the EFFECT function is used as follows:
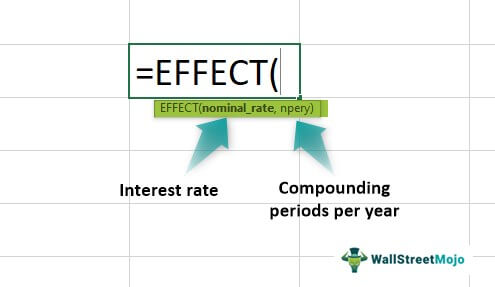
Here,
- nominal_rate is the interest rate
- npery is the number of compounding periods per year
The equation and calculation look like this:

If the nominal interest rate is 10%, compounded annually, then the Annual Equivalent Rate is the same as 10%. On the other hand, if the nominal interest rate is 10% and is compounded once in six months, the EAR derived is 10.25%.
Similarly, if the nominal interest rate of 10% is compounded quarterly, the EAR is 10.38%, and if it’s compounded monthly, the EAR is equal to 10.47%.
Example #2 - (Calculation)
Suppose Tom buys a particular instrument with the interest rate mentioned as 16%. He invests around $100,000. Moreover, the instrument is subject to compounding annually. Let us determine the EIR or EAR for this particular instrument and check how much he would get every year as interest.
The interest rate gets compounded yearly, and hence the formula is used to calculate the effective interest rate –
(1 + i/n) n – 1 = (1 + 0.16/1) 1 – 1 = 1.16 – 1 = 0.16 = 16%.
In this example, there would be no difference between the annual interest rate and an annual equivalent rate (EAR).
Every year Tom would get the interest of = ($100,000 * 16%) = $16,000 on the instrument.
Effective Interest Rate vs Nominal Interest Rate
Both effective and nominal interest rates have a vital role to play in calculating the exact returns on investment or the percentage of interest applicable on a loan. However, they share a set of differences too. Some of these differences have been listed below:
- The nominal interest rate is the one that is originally mentioned in the financial instrument or product. In contrast, the effective annual interest rate is calculated with respect to the number of compounding periods in a year.
- This makes the latter higher than its nominal counterpart.
For example, if an 11% interest rate is written on the instrument and the interest rate gets compounded four times a year, then the annual effective interest rate won’t be 11%.
It would rather be:
(1 + i/n) n – 1
= (1 + 0.11/4) 4 – 1 = 1.1123 – 1
= 0.1123 = 11.23%
Thus, in this example:
- 11 is the nominal interest rate, and
- 11.23% is the EIR for the investor or lender
Frequently Asked Questions (FAQs)
It is applicable when the nominal rates are subject to change per the number of compounding periods over a year. This is because compounding changes the interest rates, ultimately influencing investment returns or interest charges applicable to a loan. In short, the figures change as soon as the compounding occurs, be it a little change or a major one.
The EFFECT function is used to compute it in Excel. The formula is put as EFFECT (nominal_rate, npery). Here, the nominal rate is the rate mentioned in the financial instrument, and npery is the number of compounding periods per year.
The EIR is different from the Annual Percentage Rate (APR). While the former is calculated when compounding applies, the latter is computed when the rates are applied with respect to simple interest.
Effective Interest Rate Video
Recommended Articles
This article was the guide to what is Effective Interest Rate. We explain its formula, excel & calculation examples, and differences with a nominal interest rate. You may learn more from the following articles -
