Table Of Contents
Using CD Interest Calculator
A CD interest calculator will help you calculate the total amount to be received along with interest, which will be earned when you invest the amount in the certificate of deposit.
About CD Interest Calculator
The formula for calculating CD interest is as below:
M = I * ( 1 + i/N )n * N
Wherein,
- M is the total maturity amount
- I is the initial amount that is invested
- i is the fixed rate of interest
- N is the frequency of interest is paid
- n is the number of periods for which investment shall be made.
The CD is a type of investment product that stands for a certificate of deposit. This is an investment where the investor locks in his funds to earn a little higher interest rate than other products. If an investor invests for a longer period, he will earn more interest as the rate would be higher.
The interest payout could be annually, semi-annually, or quarterly, depending upon financial institution terms. The interest is compound interest, and this calculator will calculate interest accordingly and provide the result as the total amount at maturity, including interest.
The Hargreaves Lansdown provides access to a range of investment products and services for UK investors.
How To Calculate CD Interest?
One needs to follow the below steps to calculate the CD interest along with the total amount at maturity.
Step #1: Determine the initial amount which is to be invested. That would be the initial investment.
Step #2: Figure out the rate of interest that is being provided on the certificate of deposit and the frequency of the same being paid. That is how much it will be paid in a year, denoted by N.
Step #3: Now, determine the period or number of years for which it shall be invested.
Step #4: Divide the interest rate by the times the interest would be paid in a year. For example, if the rate of interest is 5% and it pays semi-annually, it means the interest would be paid out twice, and therefore the rate of interest would be 5%/2, which is 2.5%.
Step #5: Now multiply the investment amount by the applicable interest rate using the above-mentioned formula.
Step #6: The resultant figure will be the maturity amount of the certificate of deposit, including the interest.
CD Interest Examples
Example #1
JP Morgan and Chase is one of the leading investment banks in the United States. It has initiated a new product in a bucket of the certificate of deposit. The scheme states that the minimum amount which is required to be deposited is $25,000, and the minimum duration is 6 months. The APY for this scheme is 2.25% if invested for more than one year else, 1.98% for all deposits for less than a year. The interest will be compounded semi-annually.
Suppose one invests in this scheme for two years; then what will be the amount received at maturity?
Solution:
We are given the below details:
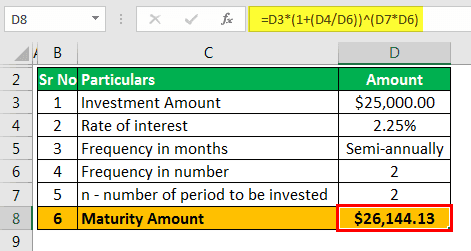
| Sr No | Particulars | Amount |
|---|---|---|
| 1 | Investment Amount | $25,000.00 |
| 2 | Rate of Interest | 2.25% |
| 3 | Frequency in Months | Semi-annually |
| 4 | Frequency in Number | 2 |
| 5 | n - number of period to be invested | 2 |
- I = $25,000
- i = Rate of interest, which is 2.25% that is applicable for a period of 2 years
- N = Frequency which is semi-annually and interest will be paid twice a year
- n = number of years the investment proposed to be made, which is 2 years here.
Now, we can use the below formula to calculate the maturity amount.
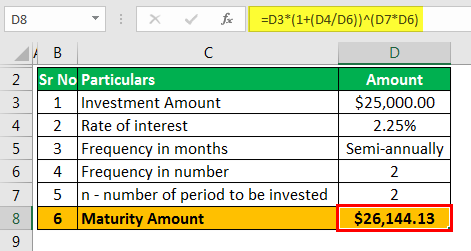
- = 25,000 * ( 1 + 2.25%/2 )2 x 2
- =$26,144.13
Compounded interest amount
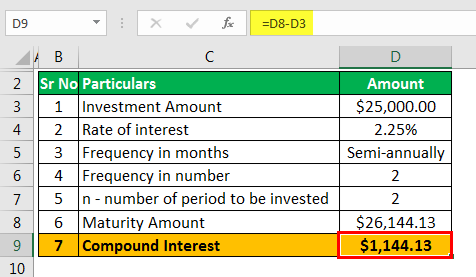
- = $26,144.13 – $25,000
- = $1,144.13
Example #2
Three banks are offering limited period CDs, and Mr. X wants to invest $89,000 in the one that pays the highest maturity amount.
| Bank I | Bank II | Bank III | |
|---|---|---|---|
| Period Allowed | 2 years | 2 years | 1 year & 6 months |
| Rate of Interest | 4.50% | 5.00% | 6.00% |
| Interest Payout | Quarterly | Annually | Semi-annually |
Based on the above information, you must advise Mr. X on where to invest to earn the maximum amount at maturity.
Solution:
BANK I
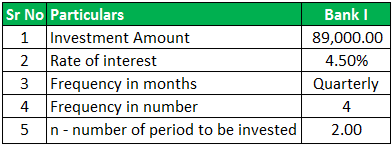
- I = $89,000
- i = Rate of interest, which is 4.50% that is applicable for a period of 2 years
- N = Frequency which is Quarterly here; hence interest payout will be 4
- n = number of years the investment to be made, which is 2 years here.
Now, we can use the below formula to calculate the maturity amount.
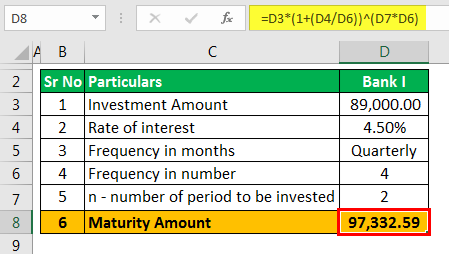
- = 89,000 x ( 1 + 4.50 / (4 x 100 ) )4 x 2
- = 97,332.59
Compounded interest amount
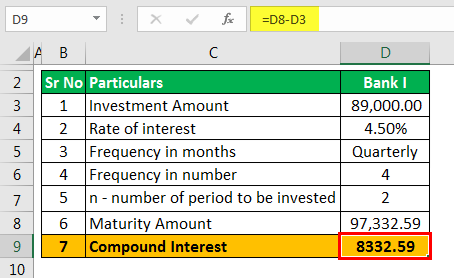
- = 97,332.59 – 89,000
- = 8,332.59
BANK II

- I = $89,000
- i = Rate of interest, which is 5.00% that is applicable for a period of 2 years
- N = Frequency which is Annually here; hence it will be 1
- n = number of years the investment to be made, which is 2 years here.
Now, we can use the below formula to calculate the maturity amount.
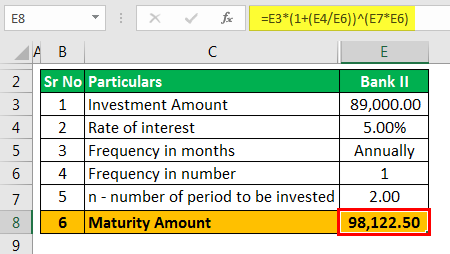
- = 89,000 x ( 1 + 5.00 / (1 x 100 ) )1 x 2
- = 98,122.50
Compounded interest amount
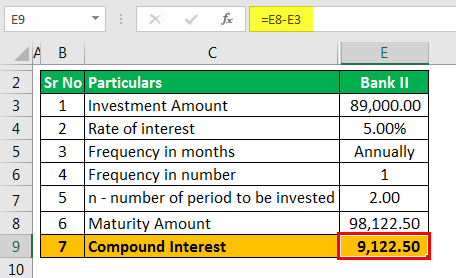
- = 98,122.50 – 89,000
- = 9,122.50
BANK III
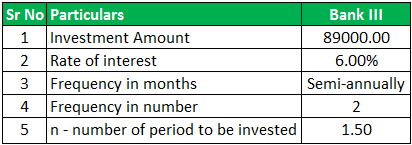
- I = $89,000
- i = Rate of interest, which is 6.00% that is applicable for a period of 1 year and 6 months
- N = Frequency which is Semi-annually here, hence it will be 6
- n = number of years the investment to be made, which is 1 year and 6 months here.
Now, we can use the below formula to calculate the maturity amount.
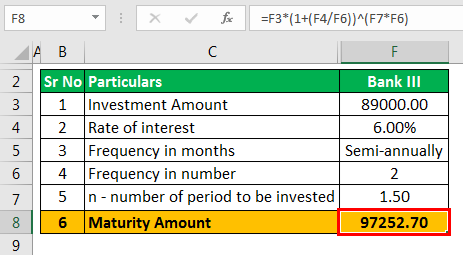
- = 89,000 x ( 1 + 6.00 / (2 x 100 ) )1.5 x 2
- = 97,252.70
Compounded interest amount

- = 97,252.70 – 89,000
- = 8,252.70
Hence, Mr. X should invest in a CD of Bank II as that is the maximum amount provided to him at maturity.
Conclusion
This calculator can calculate the maturity amount when one invests in a certificate of deposit, which offers a safer and more conservative investment than stocks and banks. There is no growth, but it offers guaranteed returns.
Disclosure: This article contains affiliate links. If you sign up through these links, we may earn a small commission at no extra cost to you.