Benford's Law

Published on :

21 Aug, 2024

Blog Author :

Edited by :

Reviewed by :

Benford's Law is a mathematical principle that displays the frequency distribution of numbers in real-life datasets. Benford's law analysis is used for detecting irregularities or fraud in data analysis. Significant deviations from the expected distribution can indicate errors, manipulation, or inaccurate reporting. This law is also known as the First-Digit Law.

According to the law, the digit 1 tends to appear as the leading digit about 30% of the time, whereas the digit 9 appears less than 5% of the time. It states that in some naturally occurring datasets, the probability of encountering a lower number as the leading digit increases as the digits increase. Conversely, the possibility of meeting a higher number as the first digit decreases.

What Is Benford's Law?

Benford's Law is a mathematical rule that observes the frequency of the leading digits in specific naturally occurring, real-life datasets. It highlights that the number 1 is the leading digit about 30% of the time, whereas the digit 9 appears less than 5%.
The law states that the probability of a lower number occurring more frequently in a dataset is higher as the numbers increase. Conversely, the possibility of a higher number appearing as the first digit decreases.
This law is used for detecting irregularities and frauds in datasets, as a significant deviation from the law in the data distribution indicates errors and misrepresentation.

Benford's Law Explained

Benford's Law, also known as the First-Digit Law, is a statistical instrument that observes the distribution of leading digits in real-life datasets. It suggests that the leading numbers are not uniformly distributed in a dataset with naturally occurring data. Instead, they follow a predictable pattern. It applies to several domains, including financial transactions, population counts, and scientific measurements. This logarithmic distribution implies that as the digits increase, the probability of encountering the higher numbers as the first digit decreases.

The underlying principle behind Benford's law analysis lies in the inherent nature of numbers in real-life data. Specific datasets display a tendency where larger numbers occur less frequently than smaller ones. However, this law is a statistical observation, not a strictly established rule. Despite its high accuracy, it cannot prove fraud or error definitively. It can be used as a tool to prompt further investigation.

Formula

Benford's law formula is as follows:

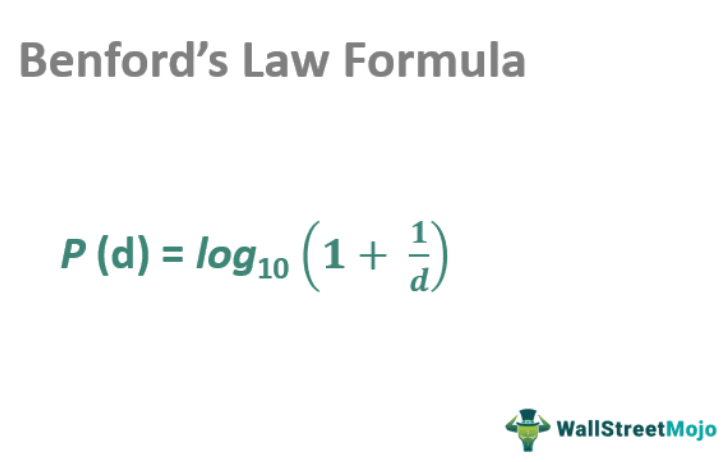
where d is the leading digit

Examples

Let us go through the following examples to understand Benford's law:

Example #1

The real-life datasets follow a pattern where the first digit of each number in a random data collection is most likely to be the minor digit, starting from 1. The design has been reflected in the number of COVID-19 cases reported worldwide. Researchers in the Journal of Public Health showed that the globally reported COVID case datasets followed the pattern. This suggested that the COVID case reporting was legitimate.

However, it did not apply to individual countries. Some countries deviated from this pattern significantly, which hinted that the COVID case numbers reported there could be fabricated or misreported. The researchers found that the suspicious data were most likely associated with less developed countries. This is an example of Benford's Law.

Example #2

Social media users have recently shared posts and graphs saying that a mathematical rule shows clear evidence of fraud in the United States presidential elections. However, the research papers and academics that Reuters consulted with said that the deviation from the rule does not clearly indicate that election fraud occurred. The posts and the graphs that compare the voting tallies show that Biden's vote tallies do not follow the law, whereas Trump's do. This is an example of Benford's Law.

Applications

Some of Benford's law applications are:

The law is commonly applicable in forensic accounting and auditing for detecting irregularities and potential fraud in financial data. If a dataset deviates significantly from the expected distribution, it can indicate manipulated or misrepresented numbers.
It serves as a tool for verifying the accuracy and integrity of large datasets. Discrepancies in datasets can be identified by comparing the observed distribution of leading digits with the expected distribution. Consequently, it could lead to further investigation and data validation.
Tax authorities use the law to identify suspicious or potentially fraudulent tax returns. Deviations from the expected digits distribution can indicate unreported income, incorrect reporting, or other tax irregularities.
The law is employed in economic studies to assess the reliability of financial data, including GDP (Gross Domestic Product), inflation rates, or economic indicators. It helps researchers and analysts identify data manipulation or errors affecting their conclusions.
This law can be used as a statistical tool for detecting potential election fraud. It can raise awareness about possible irregularities by analyzing the distribution of first digits in voting results or polling station data. As a result, fraudulent activities such as ballot stuffing or vote manipulation can be identified.
It is applied in various scientific fields, including physics, geology, and astronomy. It aids in verifying the reliability of measurement data and identifying irregularities or measurement errors that may impact research outcomes.
In image and signal processing, the law is used to detect image or signal tampering. Analyzing the distribution of leading digits in image or signal data can reveal inconsistencies that may indicate manipulation or forgery.

Limitations

The limitations of Benford's law are discussed below:

The law works more effectively when it processes large datasets. It depends on the assumption that the dataset must contain sufficient data points to estimate the expected distribution accurately. However, the law may not apply to small sample sizes with fewer data points, making its applicability less reliable.
This law assumes that the dataset results from an unbiased and organic process. If the user has intentionally manipulated or altered to deviate from or meet the expected distribution, the law may not be applicable. However, in some cases, individuals may generate fraudulent datasets precisely to mimic the anticipated law distribution, making it more challenging to detect any irregularities.
The law is a general statistical observation. It does not consider specific contextual factors that may impact the leading digits distribution. Different industries, countries, or datasets with unique characteristics may show variations from the expected distribution due to specific contextual factors.
It is primarily applicable to datasets that have numerical values. It may not be directly relevant to non-numeric or categorical data, including text, names, and qualitative variables. In these cases, alternative statistical methods are more suitable.
While the law focuses on the distribution of the first digits, it does not provide insights into the distribution of the following numbers. Other statistical tools, like the Second-Digit Law or n-Digit Laws, are more suitable for analyzing the distribution patterns beyond the first digit.
Deviations from the expected law distribution can be considered a signal for further investigation instead of definitive proof of fraud or error. The law is a statistical instrument and may not accurately represent an error or fraud. Further analysis and evidence must be applied to establish the presence of irregularities or fraudulent activities.

Frequently Asked Questions (FAQs)

1. Why does Benford's law work?

This law works because counting always begins with the lower digits or values, and they occur more frequently than the higher digits. The lower numbers must be counted first to reach the higher values. Moreover, this law works in several datasets where it is challenging to figure out why it works. In those cases, the law naturally fits and can be considered a rule of thumb.

2. When does Benford's law not apply?

The law is often not applicable for assigned numbers, like phone numbers, ID numbers, and zip codes. Additionally, even though it helps, it is unnecessary if the number displays a process involving exponential growth or a power law. However, if the value range is limited, it impacts the leading digits, and this law is less likely to apply. For instance, human characteristics, like age and height, naturally fall into limited ranges. As a result, this law does not apply to such distributions. Furthermore, restrictions imposed on potential values may also invalidate this law.

3. Does Benford's law apply to random numbers?

This law does not apply appropriately to random data sources. It applies only to the data produced by specific real-life random processes. For example, population growth is a natural process that exhibits more or less exponential growth. This process's data characterization follows the law.