Table Of Contents
Amortized Loan Definition
The amortized loan formula deals with the determination of annual or monthly payments that the borrower has to make to the lender for the loan undertaken by them. The Annual payment is composed of annual interest payments and the annual portion of the long-term debt. The interest component in the annual payment remains to be high during the initial tenure of the loan.
The annual payments could be determined using the present value of the annuity formula. The amortized payment depends upon the rate of interest, tenure of the loan, borrowed sum, and the nature of compounding as per agreement between the lenders and the borrowers.
Amortized Loans Explained
Amortized loans are a common and structured way for individuals and businesses to repay borrowed money, typically for big-ticket items like homes or vehicles. These loans involve scheduled, regular payments that cover both the principal amount borrowed and the accrued interest.
The main features and characteristics of a typical amortized loan are:
- Principal Reduction: With each installment, a portion goes toward paying down the principal amount borrowed. This means that over time, the outstanding loan balance decreases.
- Interest Payment: The remaining portion of the payment goes toward interest. In the early stages of the loan, this interest component is higher, but it gradually decreases as the outstanding balance decreases.
- Fixed Schedule: Amortized loans follow a fixed payment schedule, often monthly, where each installment is the same amount throughout the loan term. This predictability makes budgeting easier for borrowers.
- Equity Building: One significant benefit of amortized loans, especially for mortgages, is that borrowers build equity over time as they pay down the principal. This equity can be tapped into through refinancing or selling the asset.
- Interest Cost Reduction: Over the loan term, the borrower pays less in interest compared to loans with variable or interest-only payments.
Amortized loans are structured to ensure that borrowers steadily reduce their debt while repaying interest fairly. This method makes them a popular choice for long-term financing, offering financial stability and helping individuals and businesses achieve their goals without overwhelming financial burdens.
The Hargreaves Lansdown provides access to a range of investment products and services for UK investors.
Formula
The amortized loan equation can be found using the formula discussed below.
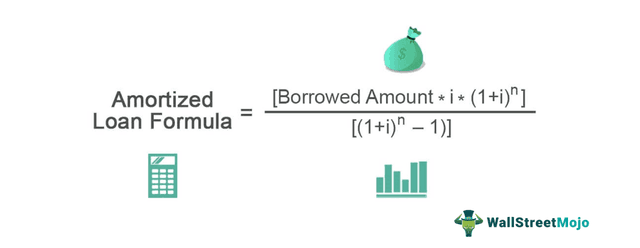
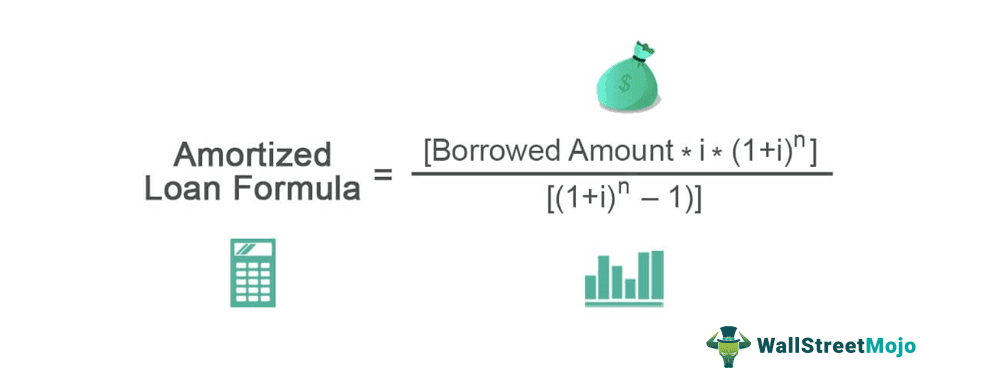
Amortized Loan Formula = / [(1+i) n – 1)
Here,
- The rate of interest is represented as i.
- The tenure of the loan is represented as n.
How To Calculate?
The amortized loan calculator can be used by using the following steps:
-
Firstly, the borrower has to determine the loan amount. The borrower citing his capacity and with the availability of loan, calculators determine the loan amount which they desire so that they can fill up the shortfall of funds.
-
Next, determine the rate of interest that the lender would be most likely to lend the amount. Basis the conditions of the economy, and as per the expectations of lenders, there could be a specific range of interest rates available to the borrowers. The borrowers have to choose the best rate of interest from the available options.
-
Next, determine the tenure of the loan, which the borrower believes he or she could service basis the paying capacity of the borrower.
-
Next, determine the product of the borrowed sum, rate of interest, and with the sum of unity and rate of interest having the power of tenure of the loan.
-
Next, deduct the sum of unity and rate of interest having the power of tenure of the loan and the unity.
-
Next, divide the resulting value from step 4 with the resulting value of step 5 to arrive at the amortized loan amount to be serviced by the borrower that the borrower has to pay to the lender to amortize the loan.
Types
Amortized loan equations come in various types, each tailored to specific financial needs and circumstances. A few of the most common types can be understood through the points below.
- Mortgages: These are long-term loans used to finance the purchase of homes. They typically have fixed interest rates and extended repayment periods, often spanning 15 to 30 years. Monthly payments cover both principal and interest, helping homeowners gradually build equity.
- Auto Loans: Auto loans finance the purchase of vehicles. They usually have shorter terms, often ranging from 36 to 72 months. Similar to mortgages, borrowers make monthly payments that reduce both the principal and interest over time.
- Personal Loans: These are versatile loans that can be used for various purposes, such as debt consolidation, home improvements, or unexpected expenses. Personal loans often have fixed interest rates and terms ranging from one to seven years.
- Student Loans: Specifically designed for education-related expenses, student loans may offer deferred payments while in school, and then borrowers make amortized payments once they graduate. They can be federal or private, with varying interest rates and terms.
- Business Loans: Entrepreneurs and businesses use these loans for capital investments, expansion, or working capital needs. Business loans can have different structures, including term loans, equipment loans, or SBA loans, all of which can be amortized.
- Installment Credit: This encompasses various types of retail financing, including credit cards with installment plans. Shoppers can pay for purchases over time with fixed monthly payments, often at lower interest rates than regular credit card balances.
Examples
Now that we understand the basics, formula, and types, let us apply the knowledge to practical application through the examples below.
Example #1
Let us take the example of a borrower who is looking for finance for his new home. There is one lender who wishes to lend a loan of $2 million with a rate of interest of 8 percent for a tenure of 20 years. Help the borrower determine the monthly amortized loan amount.
Use the following data for the calculation of Amortized Loan Amount
| Particulars | Amount |
|---|---|
| Borrowed Amount | $2,000,000 |
| Rate of Interest (i) | 8.00% |
| Time in Years (n) | 20 |
The calculation of monthly amortized loan is as follows –
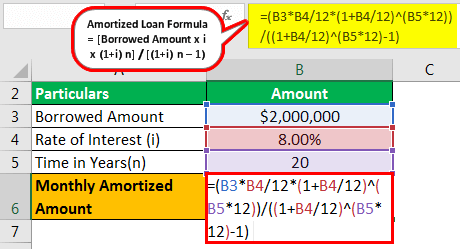
- = / /
Monthly Amortized Loan Amount will be -

- Monthly Amortized Amount = $16,728.80
If the borrower undertakes the loan, then he has to service a monthly amortized amount of $16,728.80 for the period of 20 years.
Example #2
Let us take the example of a borrower who is looking for finance for his new car. There is one lender who wishes to lend a loan of $0.5 million with a rate of interest of 8 percent for a tenure of 5 years. Help the borrower determine the monthly amortized loan amount. Determine the monthly amortized amount as displayed: -
Use the following data for the calculation of Amortized Loan Amount
| Particulars | Amount |
|---|---|
| Borrowed Amount | $500,000 |
| Rate of Interest (i) | 8.00% |
| Time in Years (n) | 5 |
The calculation of monthly amortized loan is as follows –

- = / /
Monthly Amortized Loan Amount will be -

- Monthly Amortized Amount = $ 10,138.20
If the borrower undertakes the loan, then he has to service a monthly amortized amount of $ 10,138.20 for five years.
Relevance and Use
To successfully service the loan, the borrower has to determine the amortized loan amount that he has to service throughout the tenure of the loan. Using the amortized loan calculator is considered to be advantageous if the borrower makes early payments at critical junctures to reduce the principal balance, which would, in turn, lower the high-interest payments. Before taking additional debt, it is advisable to assess the existing open accounts of loans to avoid the situation of a debt trap.
The amortized loan amount should be at least 35 percent of the annual or monthly income. Whenever the rate of interest falls, always maintain the same value of the amortized amount and reduce the tenure of the loan. This would ensure faster servicing of loans within the allocated time frame.
Amortized Loan Formula in Excel
Now, let us take the Excel example to illustrate the concept of the amortized loan in the Excel template below. The PMT function is the finance function of the excel, which helps in the determination of an amortized loan amount. The syntax of pmt is represented as follows: -
=pmt (rate, nper, pv, , )
Here,
- The rate of interest is represented as a rate.
- The number of periods is represented as nper.
- The loan amount is represented as the pv.
- The future value is represented as fv.
- The pmt value to be determined at the or beginning of the period is represented by type.
Let us consider the examples in the excel to further illustrate the concept of an amortized loan.
Example #1
Use the following data for the calculation of the Amortized Loan Amount in excel.

Calculation of Monthly Payment using the PMT function is as follows,
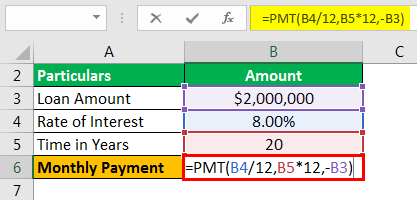
- =PMT(8.00%/12,20*12,-$2000000)
Monthly Payment will be -
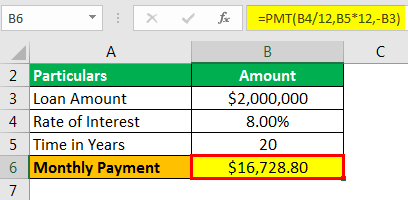
- Monthly Payment = $16,728.80
Similarly, let us consider the example 2 in the excel to further illustrate the concept of amortized loan. The table provides a detailed explanation of the risk premium.
Example #2
Use the following data for the calculation of the Amortized Loan Amount in excel.
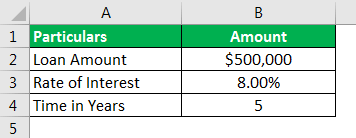
Calculation of Monthly Payment using the PMT function is as follows,
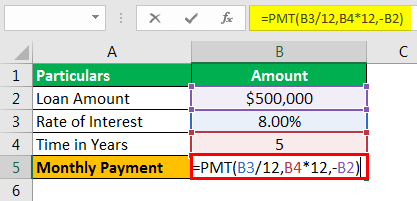
- =PMT(8.00%/12,5*12,-$500000)
Monthly Payment will be -
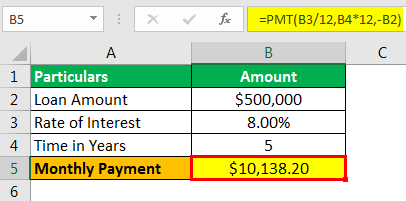
- Monthly Payment =$10138.30
Amortized Loan Vs Term Loan
The key distinction between amortized loans and term loans lies in how payments are structured. Let us understand their differences through the comparison below.
Amortized Loan
- Monthly payments cover both principal and interest.
- Over time, interest costs decrease as the principal balance reduces.
- Often used for large, long-term purchases like mortgages and auto loans.
- Payments remain constant throughout the loan term.
- Borrowers gradually build equity as they pay down the principal.
- Mortgages and auto loans are common types of amortized loans.
- May offer fixed or variable interest rates.
Term Loan
- Monthly payments primarily cover interest, with the principal paid in a lump sum at the end.
- Interest costs remain relatively high until the final payment.
- Short-term financing for businesses or individuals with specific, time-sensitive needs.
- Payments are smaller initially but balloon at the end with the principal repayment.
- No gradual equity building as in amortized loans.
- Business expansion loans or bridge loans are typical term loans.
- Can have fixed or variable interest rates.
Disclosure: This article contains affiliate links. If you sign up through these links, we may earn a small commission at no extra cost to you.