Adjusted Present Value

Adjusted present value refers to a method used in valuing firms and projects by totaling the NPV of a firm or project, assuming that it is based on equity financing and the present value of financing side effects.

The method was developed by Stewart Myers, a financial economics professor. The approach portrays the value of a levered firm or project as the sum of the value obtained by anticipating it as an unlevered firm or project and side effects due to leverages like debt. When used in combination with other new valuation methods, it functions as an appropriate configuration for studying issues in corporate valuation.

• The adjusted present value (APV) approach portrays the value of a leveraged firm or project as the sum of NPV of the value of the firm or project like it is an unleveraged and side effect of leverages. 
• It is used in the corporate valuation of projects and firms.
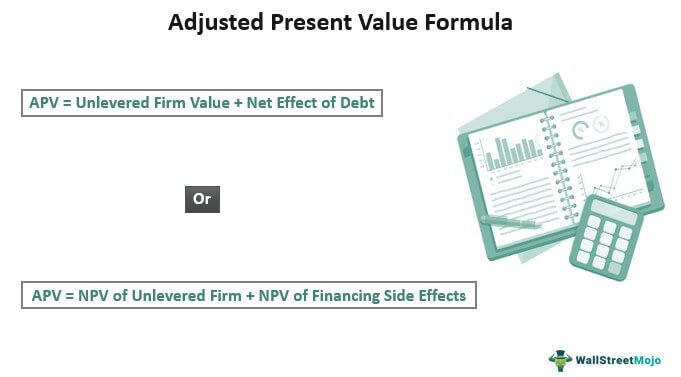
• The APV formula: APV = Unlevered Firm Value + Net Effect of Debt or APV= NPV of unlevered firm + NPV of financing side effects.
• The APV helps companies understand the importance of financing side effects.

The adjusted present value method is considered flexible, straightforward, and transparent. It can accommodate numerous financing components of complex projects, special situations, or various investment and financing effect interactions. Professional users of the APV formula can refine it according to the specific scenario involved. For example, it is appropriate for valuing International projects or projects involving FDIs since the APV framework can incorporate the special financial considerations associated with the valuation of foreign projects.

Many academics perceive it as a superior method compared to other valuation techniques. Compared to the Weighted Average Cost of Capital (WACC) valuation method, the APV approach demonstrates benefits that make it more appealing. APV approach easily confronts complex situations like fluctuating capital structures, dividend policies, and pension liabilities. It functions well for complex projects and transactions like leveraged buyouts. It segregates and values relevant components like valuing all kinds of financing side effects.

In contrast, WACC is not as flexible as the APV method in separately valuing the financing side effects. Also, the APV method does not necessitate the restrictive or stringent assumptions of WACC. Furthermore, WACC is more susceptible to major errors than APV.

The APV method involves calculations for different components and ultimately points to the firm's or project's value. A positive APV is always favorable compared to a negative APV. The approach interprets how the debt influences a firm's project's value.

The general adjusted present value formula used for calculation is:

APV = Unlevered Firm Value + Net Effect of Debt

or

APV= NPV of Unlevered Firm + NPV of Financing Side Effects.

Important elements of the approach are the unlevered cost of equity, the expected future capital structure, expected future free cash flows, financing side effects, etc. Some of the financing side effects are interest tax shields, subsidies, hedges, and issue costs. If the calculation of the unlevered cost of equity is difficult to discern, it can be obtained from the levered cost of equity for the firm or a comparable entity. Similarly, if the tax shield discount rate is unknown, a rate larger than or comparable to the cost of debt and less than or identical to the unlevered cost of equity is selected.

Let's interpret the APV method using a simple example. The net present value of the unlevered firm is $200,000 (PV of unleveraged cash flows obtained by discounting the FCF forecasted based on the un-leveraged cost of equity). The applicable tax rate is 35%, the debt level is $70,000, and the interest rate is 5%. Now calculate the adjusted present value of the project.

Given,

• The PV of unlevered firm is $200,000
• Tax rate: 35%
• Debt: $70,000
• Interest rate: 5%

Interest tax shield: (Debt load*interest rate*tax rate)/interest rate

= (70,000*5%*35%)/5%

=$24,500

APV= Unlevered Firm Value + Net Effect of Debt

=$200,000+$24,500

=$224,500

This has been a Guide to What is Adjusted Present Value (APV) Method of Valuation. We explain the APV approach using its formula and examples. You may also have a look at the following articles to learn more –

• Net Present Value (NPV)
• Real Estate Valuation
• Valuation Methods